**Section III. VALUE
Chapter 26. VALUE AND CORPORATE FINANCE
Chapter 26
VALUE AND CORPORATE FINANCE
No, Sire, it’s a revolution!
This section presents the concepts and theories that underpin all important financial decisions. In particular, we will examine their impact on value, keeping in mind that basically to maximise a value, we must minimise a cost. The chapters in this section will introduce you to the investment decision processes within a firm and their impact on the overall value of the company.
Section 26.1 THE FINANCIAL PURPOSE OF A COMPANY IS TO CREATE VALUE
1/ INVESTMENT AND VALUE
The accounting rules we looked at in Chapter 4 showed us that an investment is a use of funds, but not a reduction in the value of assets. We will now go one step further and adopt the viewpoint of the financial manager for whom a sufficiently profitable investment is one that increases the value of capital employed.
We shall see that a key element in the theory of markets in equilibrium is the market value of capital employed. This theory underscores the direct link between the return on a company’s investments and that required by investors buying the financial securities issued by the company.
The true measure of an investment policy is the effect it has on the value of capital employed. This concept is sometimes called “enterprise value”, a term our reader should not confuse with the value of equity (capital employed less net debt). The two are far from the same!
Hence the importance of every investment decision, as it can lead to three different outcomes:
- Where the expected return on an investment is higher than that required by investors, the value of capital employed rises instantly. An investment of 100 that always yields 15% in a market requiring a 10% return is worth 150 (100 × 15% / 10%). The value of capital employed thus immediately rises by 50.
- Where the expected return on the investment is equal to that required by investors, there is neither gain nor loss. The investors put in 100, the investment is worth 100 and no value has been created.
- Where the expected return on an investment is lower than that required by investors, they have incurred a loss. If, for example, they invested 100 in a project that in the end should only yield 6% to perpetuity, then the value of the project is only 60 (100 × 6% / 10%), giving an immediate loss in value of 40.
- Value remains constant if the expected rate of return is equal to that required by the market.
- An immediate loss in value results if the return on the investment is lower than that required by the market.
- Value is effectively created if the expected rate of return is higher than that required by the market.
The resulting gain or loss is simply the positive or negative net present value that must be calculated when valuing any investment. All this means, in fact, is that if the investment was fairly priced, then nothing changes for the investor. If it was “too expensive”, the investors take a loss, but if it was a good deal, they earn a profit.
The graph below shows that value is created (the value of capital employed exceeds its book value) when return on capital employed exceeds the weighted average cost of capital, i.e. the rate of return required by all suppliers of funds to the company.
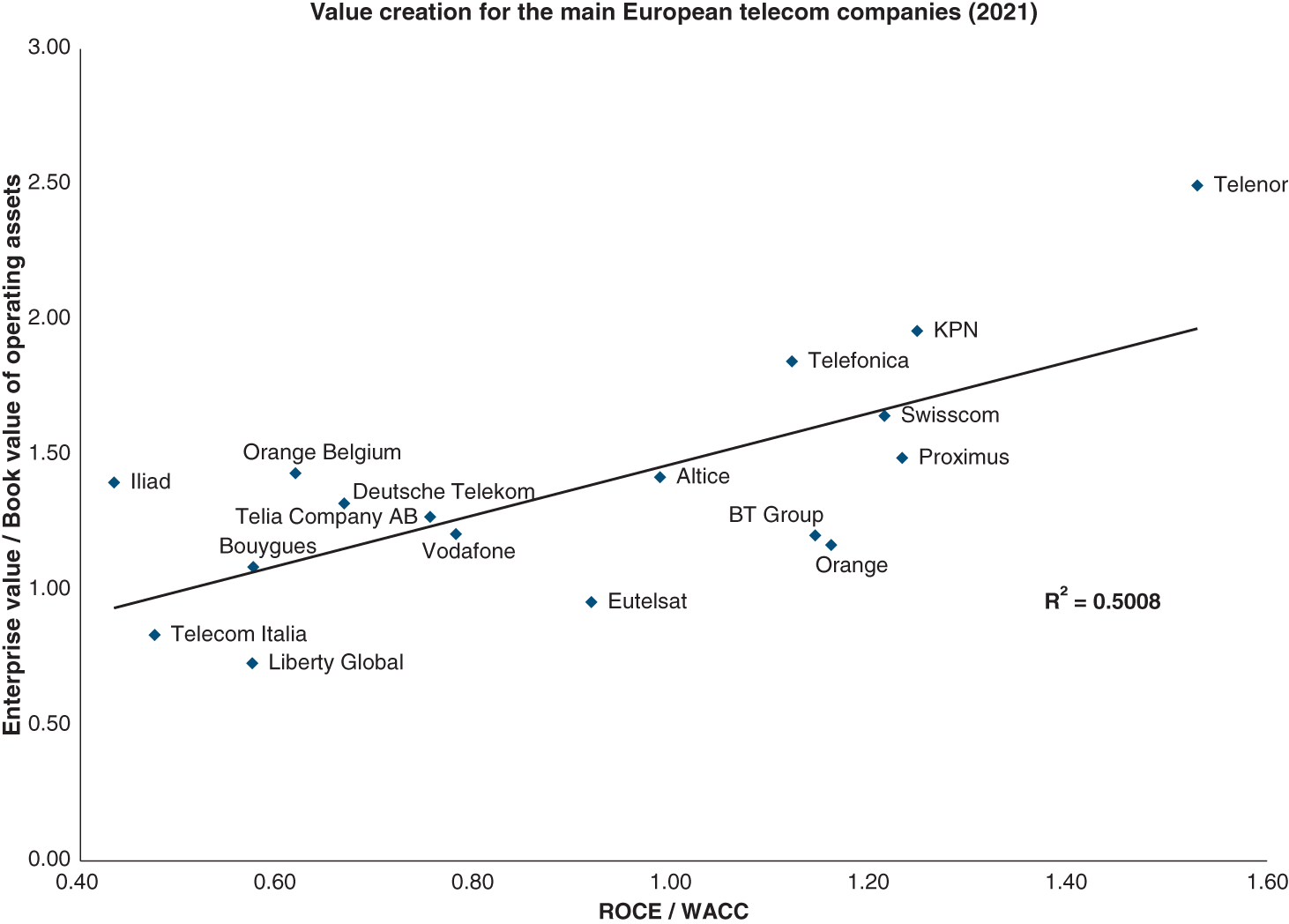
Source: Data from Exane BNP Paribas
2/ THE RELATIONSHIP BETWEEN COMPANIES AND THE FINANCIAL WORLD
In the preceding chapters we examined the various financial securities that make up the debt issued by a company from the point of view of the investor. We shall now cross over to the other side to look at them from the issuing company’s point of view.
- Each amount contributed by investors represents a resource for the company.
- The financial securities held by investors as assets are recorded as liabilities in the company’s balance sheet.
- And, most importantly, the rate of return required by investors represents a financial cost to the company.
At the financial level, a company is a portfolio of assets financed by the securities issued on financial markets. Its liabilities, i.e. the securities issued and placed with investors, are merely a financial representation of the industrial or operating assets. The financial manager’s job is to ensure that this representation is as transparent as possible.
What is the role of the investor?
Investors play an active role when securities are issued, because they can simply refuse to finance the company by not buying the securities. In other words, if the financial manager cannot come up with a product offering a risk/reward trade-off acceptable to investors, then the lack of funding will eventually push the company into bankruptcy.
We shall see that when this happens, it is often too late. However, the financial system can impose a sanction that is far more immediate and effective: the valuation of the securities issued by the company.
Financial markets continuously value the securities in issue. In the case of debt instruments, rating agencies assign a credit rating to the company, thus determining the value of its existing debt and the terms of future loans. Similarly, by valuing the shares issued the market is, in fact, valuing the company’s equity.
So how does this mechanism work?
If a company cannot satisfy investors’ risk/reward requirements, it is penalised by a lower valuation of its capital employed and, accordingly, its equity. Suppose a company offers the market an investment of 100 that is expected to yield 10 every year over a period long enough to be considered to perpetuity.1 However, the actual yield is only 5 and shows no sign of improvement. The disappointed investors who were expecting a 10% return will try to get rid of their investment. The equilibrium price will be 50, because at this price investors receive a return of 10% (5 / 50) and it is no longer in their interests to sell. But by now it is too late.
Investors who are unhappy with the offered risk/reward trade-off sell their securities, thus depressing the value of the securities issued and of capital employed, since the company’s investments are not profitable enough with regard to their risk. True, the investor takes a hit, but it is sometimes wiser to cut one’s losses.
In doing so, he is merely giving tit for tat: an unhappy investor will sell off his securities, thus lowering prices. Ultimately, this can lead to financing difficulties for the company, and increased dilution for the shareholder.
The “financial sanction” affects first and foremost the valuation of the company via the valuation of its shares and debt securities.
As long as the company is operating normally, its various creditors are fairly well protected.2 Most of the fluctuation in the value of its debt stems from changes in interest rates, so changes in the value of capital employed derive mainly from changes in the value of equity. We see why the valuation of equity is so important for any normally developing company. This does not apply just to listed companies: unlisted companies are also affected whenever they envisage divestments, alliances, transfers or capital increases.
The role of creditors looms large only when the company is in difficulty. The company then “belongs” to the creditors, and changes in the value of capital employed derive from changes in the value of the debt, by then generally lower than its nominal value. This is where the creditors come into play.
3/ IMPLICATIONS
Since we consider that creating value is the overriding financial objective of a company, it follows that:
- A financial decision harms the company if it reduces the value of capital employed.
- A decision is beneficial to the company if it increases the value of capital employed.
A word of caution, however! Contrary to appearances, this does not mean that every good financial decision increases earnings or reduces costs.
Remember, we are not in the realm of accounting, but in that of finance – in other words, value. An investment financed by cash from operations may increase earnings, but could still be insufficient with regard to the return expected by the investor who, as a result, has lost value.
Certain legal decisions, such as restricting a shareholder’s voting rights, have no immediate impact on the company’s earnings or cash, yet may reduce the value of the corresponding financial security and thus prove costly to the holder of the security.
We cannot emphasise this aspect enough, and insist that you adopt this approach before immersing yourselves further in the raptures of financial theory.
Section 26.2 VALUE CREATION AND MARKETS IN EQUILIBRIUM
1/ A CLEAR THEORETICAL FOUNDATION
We have just said that a company is a portfolio of assets and liabilities, and that the concepts of cost and revenue should be seen within the overall framework of value. Financial management consists of assessing the value created for the company’s fund providers.
Can the overall value of the company be determined by an optimal choice of assets and liabilities? If so, how can you be sure of making the right decisions to create value?
You may already have raised the following questions:
- Can the choice of financing alone increase the value of the firm? Is capital employed financed half by debt and half by equity worth more than if it were financed wholly through equity?
- Can the entrepreneur increase the value of capital employed – that is, influence the market’s valuation of it – by either combining independent industrial and commercial investments or implementing a shrewd financing policy?
If your answer to all these questions is yes, you attribute considerable powers to financial managers. You consider them capable of creating value independently of their industrial and commercial assets.
And yet, the equilibrium theory of markets is very clear:
We now provide a more formal explanation of the above rule, which is based on arbitrage.
To this end, let us simplify things by imagining that there are just two options for the future: either the company does well or it does not. We shall assign an equal probability to each of these outcomes.
We shall see how the free cash flow of three companies varies in our two states of the world:
FREE CASH FLOW
| State of the world: bad | State of the world: good | |
|---|---|---|
| A | 200 | 1,000 |
| B | 400 | 500 |
| G | 600 | 1,500 |
Note that the sum of the free cash flows of companies A and B is equal to that of company G. We shall demonstrate that the share price of company G is equal to the sum of the prices of shares of B and A.3 To do so, let us assume that this is not the case, and that VA + VB > VG (where VA, VB and VG are the respective share prices of A, B and G).
You will see that no speculation is necessary here to earn money. Taking no risk, you sell short one share of A and one share of B and buy one share of G. You immediately receive VA + VB − VG > 0; yet, regardless of the company’s fortunes, the future negative flows of shares of A and B (sold) and positive flows of shares of G (bought) will cancel each other out. You have realised a gain through arbitrage.
The same method can be used to demonstrate that VA + VB < VG is not possible in a market that is in equilibrium. We therefore deduce that VA + VB = VG. It is thus clear that a diversified company, in our case G, is not worth more than the sum of its two divisions A and B.
Let us now look at the following three securities:
FREE CASH FLOW
| Company | State of the world: bad | State of the world: good |
|---|---|---|
| C | 100 | 1,000 |
| D | 500 | 500 |
| E | 600 | 1,500 |
According to the rule demonstrated above, VC + VD = VE. Note that security D could be a debt security and C share capital. E would then be the capital employed. The value of capital employed of an indebted company (V(C+D)) can be neither higher nor lower than that of the same company if it had no debt (VE).
The additivity rule is borne out in terms of risk: if the company takes on debt, then financial investors can stabilise their portfolios by adding less risky securities. Conversely, they can go into debt themselves in order to buy less risky securities. So why should they pay for an operation they can carry out themselves at no cost?
This reasoning applies to diversification as well. If its only goal is to create financial value without generating industrial and commercial synergies, then there is no reason why investors should entrust the company with the diversification of their portfolio.
2/ ILLUSTRATION
Are some asset combinations worth more than the value of their individual components, regardless of any industrial synergies arising when some operations are common to several investment projects? In other words, is the whole worth more than the sum of its parts (2 + 2 = 5)?
Or again, is the required rate of return lower simply because two investments are made at the same time?
Company managers are fuzzy on this issue. They generally answer in the negative, although their actual investment decisions tend to imply the opposite. Take Prisma (a publisher of magazines), for example, which was bought by Vivendi in 2021. If financial synergies exist, one would have to conclude that the required rate of return in the magazine publishing industry differs depending on whether the company is independent or part of a group. Prisma would therefore appear to be worth more as part of the Vivendi group than on a standalone basis.
The question is not as specious at it seems. In fact, it raises a fundamental issue. If the required return on Prisma has fallen since it became part of Vivendi, its financing costs will have declined as well, giving it a substantial, permanent and possibly decisive advantage over its competitors.
Diversifying corporate activities reduces risk, but does it also reduce the rate of return required by investors?
Suppose the required rate of return on a company producing a single product is 10%. The company decides to diversify by acquiring a company of the same size on which the required rate of return is 8%. Will the required rate of return on the new group be lower than (10% + 8%) / 2 = 9% because it carries less risk than the initial single-product company?
We must not be misled into believing that a lower degree of risk must always be matched by a lower required rate of return. On the contrary: markets only remunerate systematic or market risks, i.e. those that cannot be eliminated by diversification. We have seen that unsystematic or specific risks, which investors can eliminate by diversifying their portfolios, are not remunerated. Only non-diversifiable risks related to market fluctuations are remunerated. This point was discussed in Chapter 18.
Since diversifiable risks are not remunerated, a company’s value remains the same whether it is independent or part of a group. Prisma is not worth more now that it has become a division of Vivendi. All else being equal, the required rate of return in the robot sector is the same whether the company is independent or belongs to a group.
On the other hand, Prisma’s value will increase if, and only if, Vivendi’s management allows it to improve its return on capital employed.
Value is created only when the sum of cash flows from the two investments is higher because they are both managed by the same group. This is the result of industrial synergies (2 + 2 = 5), and not financial synergies, which do not exist.
The large groups that indulged in a spate of financial diversifications in the 1960s have since realised that these operations were unproductive and frequently loss-making. Diversification is a delicate art that can only succeed if the diversifying company already has expertise in the new business. Combining investments per se does not maximise value, unless industrial synergies exist. Otherwise, an investment is either “good” or “bad” depending on its return compared to the required rate of return.
In other words, managers must act on cash flows; they cannot influence the discount rate applied to them unless they reduce their risk exposure.
3/ A FIRST CONCLUSION
The value of the securities issued by a company is not connected to the underlying financial engineering. Instead, it simply reflects the market’s reaction to the perceived profitability and risk of the industrial and commercial operations.
The equilibrium theory of markets leads us to a very simple and obvious rule, that of the additivity of value, which in practice is frequently neglected. Regardless of developments in financial criteria, in particular earnings per share, value cannot be created simply by adding (diversifying) or reducing value that is already in equilibrium.
We emphasise that this rule applies to listed and unlisted companies alike, a fact that the latter are forced to face at some point. Capital employed always has an equilibrium value, and the entrepreneur must ultimately recognise it.
This approach should be incorporated into the methodology of financial decision-making. Some strategies are based on maximising other types of value, for example the capability to cause harm to competitors. They are particularly risky and are outside the conceptual framework of corporate finance.
Does this mean that, ultimately, financing or diversification policies have no impact on value?
Our aim is not to encourage nihilism, merely a degree of humility.
Section 26.3 VALUE AND ORGANISATION THEORIES
1/ LIMITS OF THE EQUILIBRIUM THEORY OF MARKETS
The equilibrium theory of markets offers an overall framework, but it completely disregards the immediate interests of the various parties involved, even if their interests tend to converge in the medium term.
Since the equilibrium theory demonstrates that finance cannot change the size of the capital employed, but only how it is divided up, it follows that many financial problems stem from the struggle between the various players in the financial realm.
First and foremost we have the various parties providing funding to the company. To simplify matters, they can be divided into two categories: shareholders and creditors. But we shall soon see that, in fact, each type of security issued gives rise to its own interest group: shareholders, preferred creditors, ordinary creditors, subordinated creditors, investors in convertible bonds, etc. Further on in this chapter, we shall see that interests may even diverge within the same funding category.
One example should suffice. According to the equilibrium theory of markets, investing at the required rate of return does not change the value of capital employed. But if the investment is very risky and, therefore, potentially very profitable, creditors, who earn a fixed rate, will only see the increased risk without a corresponding increase in their return. The value of their claims thus decreases, to the benefit of shareholders whose shares increase by the same amount, the value of capital employed remaining the same. And yet, this investment was made at its equilibrium price.
This is where the financial manager comes into play! Their role is to distribute value between the various parties involved. In fact, the financial manager must be a negotiator at heart.
But let’s not forget that the managers are stakeholders as well. Since portfolio theory presupposes good diversification, there is a distinction between investors and managers, who have divergent interests with different levels of information (internal and external). This last point calls into question one of the basic tenets of the equilibrium theory, which is that all parties have access to the same information (see Chapter 15).
2/ SIGNALLING THEORY AND ASYMMETRIC INFORMATION
Signalling theory is based on two basic ideas:
- the same information is not available to all parties: the managers of a company always have more information than investors;
- even if the same information were available to all, it would not be perceived in the same way, a fact frequently observed in everyday life.
Thus, it is unrealistic to assume that information is fairly distributed to all parties at all times, i.e. that it is symmetrical as in the case of efficient markets. On the contrary, asymmetric information is the rule.
This can clearly raise problems. Asymmetric information may lead investors to undervalue a company. As a result, its managers might hesitate to increase its capital because they consider the share price to be too low. This may mean that profitable investment opportunities are lost for lack of financing, or that the existing shareholders find their stake adversely diluted because the company has launched a capital increase anyway.
This is where the communication policy comes into its own. Basing financial decisions on financial criteria alone is not enough: managers also have to convince the markets that these decisions are wise.
The cornerstone of the financial communications policy is the signal the managers of a company send to investors.
Contrary to what many financial managers and CEOs believe, the signal is neither an official statement nor a confidential tip. It is a real financial decision, taken freely and which may have negative financial consequences for the decision-maker if it turns out to be wrong.
After all, investors are far from naive and they take each signal with the requisite pinch of salt. Three points merit attention:
- Investors’ first reaction is to ask themselves why the signal is being sent, since nothing comes for free in the financial world. The signal will be perceived negatively if the issuer’s interests are contrary to those of investors. For example, the sale of a company by its majority shareholder would, in theory, be a negative signal for the company’s growth prospects. Managers must therefore persuade the buyer of the contrary or provide a convincing explanation for the disposal.
Similarly, owner-managers cannot fool investors by praising the merits of a capital increase without subscribing to it!
However, the market will consider the signal to be credible if it deems that it is in the issuer’s interest that the signal be correct. This would be the case, for example, if the managers reinvest their own assets in the company (the signal is even greater if the entrepreneur takes debt to invest).
- The reputation of management and its communications policy certainly play a role, but we must not overestimate their importance or lasting impact.
- The market supervisory authorities stand ready to impose penalties on the dissemination of misleading information or insider trading. If investors, particularly international investors, believe that supervision is effective, they will factor this into their decisions. That said, some managers may be tempted to send incorrect signals in order to obtain unwarranted advantages. For example, they could give overly optimistic guidance on their company’s prospects in order to push up share prices. However, markets catch on to such misrepresentations quickly and react to incorrect signals by piling out of the stock.
In such a context, the “watchdog” role played by the market authorities is crucial and the recent past has shown that the authorities intend to assume it in full. Such rigour is essential if we are to have the best possible financial markets and the lowest possible financing costs for companies.
Financial managers must therefore always consider how investors will react to their financial decisions. They cannot content themselves with wishful thinking, but must make a rational and detailed analysis of the situation to ensure that their communication is convincing.
Signalling theory says that corporate financial decisions (e.g. financing, dividend payout) are signals sent by the company’s managers to investors. It examines the incentives that encourage good managers to issue the right signals and discourage managers of ailing companies from using these same signals to give a misleading picture of their company’s financial health.
- investments are not maximised because the cost of financing is too high;
- the choice of financing is skewed in favour of sources (such as debt) where there is less information asymmetry.
Stephen Ross initiated the main studies in this field in 1977.
3/ AGENCY THEORY
Agency theory says that a company is not a single, unified entity. It considers a company to be a legal arrangement that is the culmination of a complex process in which the conflicting objectives of individuals, some of whom may represent other organisations, are resolved by means of a set of contractual relationships.
On this basis, a company’s behaviour can be compared to that of a market, insofar as it is the result of a complex balancing process. Taken individually, the various stakeholders in the company have their own objectives and interests that may not necessarily be spontaneously reconcilable. As a result, conflicts may arise between them, especially since our modern corporate system requires that the suppliers of funds entrust the managers with the actual administration of the company.
Agency theory analyses the consequences of certain financial decisions in terms of risk, profitability and, more generally, the interests of the various parties. It shows that some decisions may go against the simple criteria of maximising the wealth of all parties to the benefit of just one of the suppliers of funds.
To simplify, we consider that an agency relationship exists between two parties when one of them, the agent, carries out an activity on behalf of the other, the principal. The agent has been given a mandate to act or take decisions on behalf of the principal. This is the essence of the agency relationship.
This very broad definition allows us to include a variety of domains, such as the resolution of conflicts between:
- executive shareholders/non-executive shareholders;
- non-shareholder executives/shareholders;
- creditors/shareholders.
Shareholders give the company executives a mandate to manage to the best of their ability the funds that have been entrusted to them. However, their concern is that the executives could pursue objectives other than maximising the value of the equity, such as increasing the company’s size at the cost of profitability, minimising the risk to capital employed by rejecting certain investments that would create value but could put the company in difficulty if they fail, etc.
One way of resolving such conflicts of interest is to use stock options, performance shares (shares offered to management depending on certain targets being reached) or free shares, thus linking management compensation to share performance (see Chapter 43).
Debt plays a role as well, since it has a constraining effect on managers and encourages them to maximise cash flows so that the company can meet its interest and principal payments. Failing this, the company risks bankruptcy and the managers lose their jobs. Maximising cash flows is in the interests of shareholders as well, since it raises the value of shareholders’ equity. Thus, the interests of management and shareholders converge. Maybe debt is the modern whip! This is sometimes referred to as “the discipline of debt”.
The diverging interests of the various parties generate a number of costs called “agency costs”. These comprise:
- the cost of monitoring managers’ efforts (control procedures, audit systems, performance-based compensation) to ensure that they correspond to the principal’s objectives. Stock options and free shares represent an agency cost since they are exercised at less than the going market price for the stock;
- the costs incurred by the agents to vindicate themselves and reassure the principals that their management is effective, such as the publication of annual reports, the organisation of regular meetings with investors;
- residual costs.
The main references in this field are Jensen and Meckling (1976), Grossman and Hart (1980) and Fama (1980). Their research aimed to provide a scientific explanation of the relationship between managers and shareholders and its impact on corporate value.
This research forms the intellectual foundation on which the concept of corporate governance was built (see Chapter 43).
4/ FREE RIDERS
We saw above that the interests of the different types of providers of funds may diverge, but so may those of members of the same category.
This means, first, that there must be several – usually a large number – of investors in the same type of security and, second, that a specific operation is undertaken implying some sort of sacrifice, at least in terms of opportunity cost, on the part of the investors in these securities.
As a result, when considering a financial decision, one must examine whether free riders exist and what their interests might be.
Below are two examples:
- Responding to a takeover bid: if the offer is motivated by an expectation of synergies between the bidding company and its target, then the business combination will create value. This means that it is in the general interest of all parties for the bid to succeed and for the shareholders to tender their shares. However, it would be in the individual interest of these same shareholders to hold on to their shares in order to benefit fully from the future synergies.
- Bank A holds a small claim on a cash-strapped company that owes money to many other banks. It would be in the interests of the banks as a whole to grant additional loans to tide the company over until it can pay them back, but the interest of our individual bank would be to let the other banks, which have much larger exposure, advance the funds themselves. Bank A would thus hold a better-valued existing claim without incurring a discount on the new credits granted.
Section 26.4 HOW CAN WE CREATE VALUE?
Before we begin simulating different rates of return, we would like to emphasise once again that a project, investment or company can only realise extraordinary returns if it enjoys a strategic advantage. The equilibrium theory of markets tells us that under perfect competition, the net present value of a project should be nil. If a financial manager wants to advise on investment choices, he will no doubt have to make a number of calculations to estimate the future return of the investment. But he will also have to look at it from a strategic point of view, incorporating the various economic theories he has learned.
A project’s real profitability can only be explained in terms of economic rent – that is, a position in which the return obtained on investments is higher than the required rate of return given the degree of risk. The essence of all corporate strategies is to obtain economic rents – that is, to generate imperfections in the product market and/or in factors of production, thus creating barriers to entry that the corporate managers strive to exploit and defend.
But don’t fool yourself, economic rents do not last forever. Returns that are higher than the required rate, taking into account the risk exposure, inevitably attract the attention of competitors (Tesla) or of the antitrust authorities, as in the case of Google. Sooner or later, deregulation and technological advances put an end to them. There are no impregnable fortresses, only those for which the right angle of attack has not yet been found.
A strategic analysis of the company is thus essential to put the figures in their economic and industrial context, as we explained in Chapter 8.
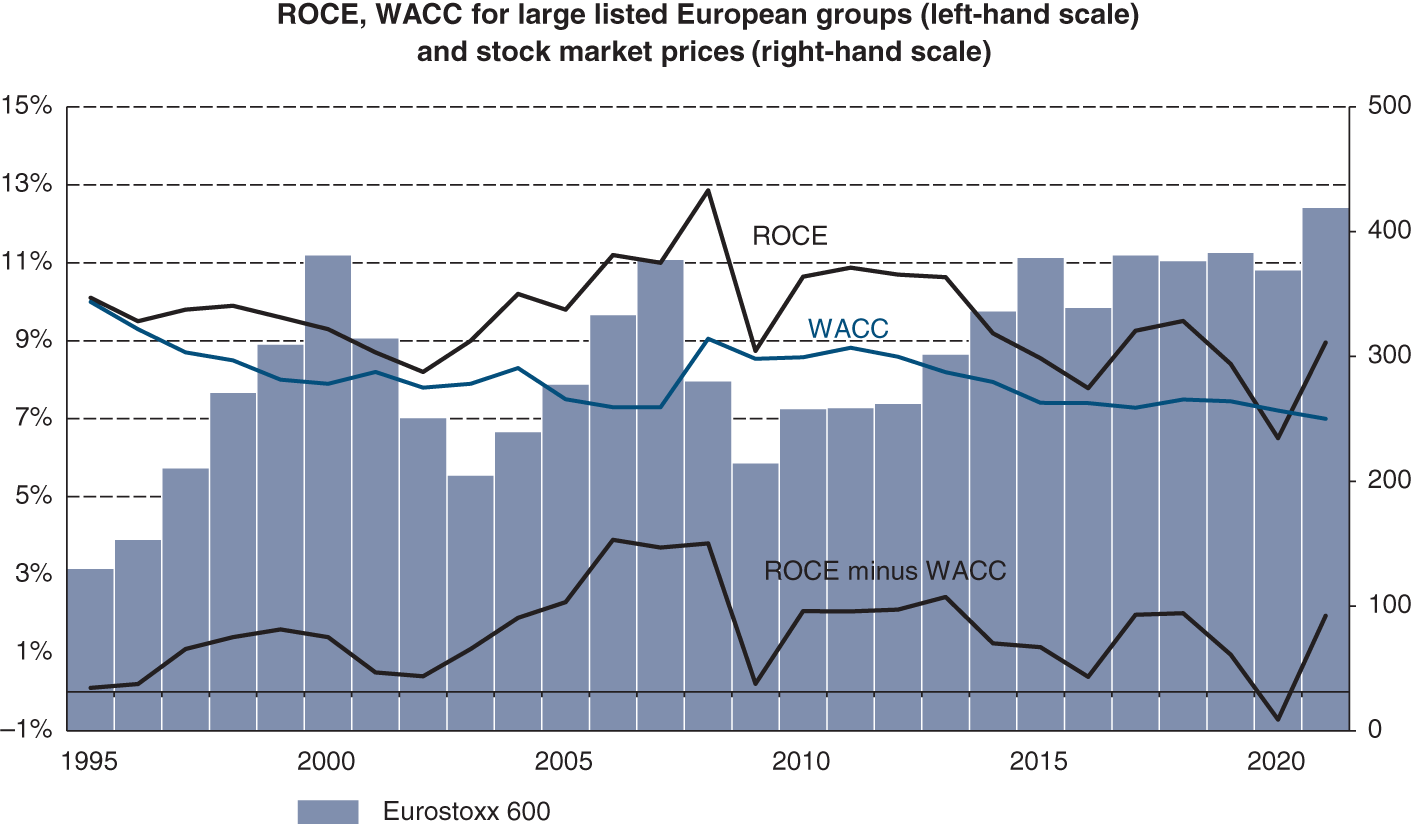
Source: Exane BNP Paribas, Factset
We insist on the consequences of a good strategy. When based on accurate forecasts, it immediately boosts the value of capital employed and, accordingly, the share price. This explains the difference between the book value of capital employed and its market value.
Rather than rising gradually as the returns on the investment accrue, the share price adjusts immediately so that the investor receives the exact required return, no more, no less. And if everything proceeds smoothly thereafter, the investment will generate the required return until expectations prove too optimistic or too pessimistic.
Section 26.5 VALUE AND TAXATION
Depending on the company’s situation, certain types of securities may carry tax benefits. You are certainly aware that tax planning can generate savings, thereby creating value or more likely preventing the loss of value. Reducing taxes is a form of value creation for investors and shareholders. All else being equal, an asset with tax-free flows is worth more than the same asset subject to taxation.
Better to have a liability with cash outflows that can be deducted from taxes than the same liability with outflows that are not deductible.
This goes without saying, and any CFO will do their best to reduce tax payments, while conforming with the spirit (and not just the strict letter) of the law. Society as a whole is no longer as forgiving of irresponsible behaviour in this domain.
They must carefully examine the impact each financial decision will have on taxes.
Our experience tells us that taking a financial decision solely on the basis of tax considerations is rarely the right thing to do. The failure of the AstraZeneca–Pfizer attempted merger, which was mainly based on fiscal considerations, is a good illustration of this.
Section 26.6 A BREAK BEFORE MOVING TO THE NEXT STEP
This chapter is dense and we would advise readers who may be discovering this information for the first time, to reread and meditate on it.
They may have been surprised or shocked to see us define value creation as the financial objective of the company, so much so that this term, which has been popular since the early 1990s, has become a mandatory part of the discourse of most managers, sometimes giving the impression that it is just management speak.
Value creation is not synonymous, as it is sometimes hastily accused of being, with redundancies, plant closures, cost cutting, and neglect of the environment, labour laws and human dignity. Quite the contrary! When we look at the list of groups that have created lasting value for their shareholders, often over very long periods, we immediately see that these are companies that have constantly innovated, grown, created markets, responded to new needs, hired and trained staff, built loyalty, created strong ties with their customers – such as Dassault Systemes, Apple, Tesla, SEB, BMW and so on.
A cost-cutting strategy can only be temporary. It cannot create lasting shareholder value unless it quickly leads to a strategy of profitable growth (as Kraft Heinz has unwillingly demonstrated).
Creating value means avoiding destroying it. In other words, avoiding bad decisions, avoiding the loss through waste of part of a scarce resource which you have been entrusted with, equity, which could have been better allocated elsewhere, and for the benefit of the many. One should avoid penalising personal savings, which have taken risks.
As we have said, and this is one of the main concepts of this chapter, the financial manager thinks in terms of value. Not in cost or profit. In value.
And that changes everything.
Because value encompasses all the financial consequences of decisions, in the short, medium and long term. The financial manager is not there to make a quick deal as a trader would. The long-term consequences of the decisions she takes today do not normally leave her indifferent, because they have an immediate impact on value. This is an impact that can be mitigated by the mechanics of discounting, but an impact nonetheless.
The financial manager, by joining a company, does not abandon her status as a citizen. To each their role and responsibilities however. In today’s environment, the role of the State is to encourage companies, through regulations and taxation, to adopt virtuous behaviour in terms of ecological transition, social responsibility and sustainability. The role of the company is to adapt its strategy, processes and procedures in order to follow that road. The role of the financial manager is to ensure that the commitments made in these and other areas are respected.
It goes without saying that a company may voluntarily be a leader in these areas and do more than is required by regulations. For example, L’Oréal guarantees all its employees worldwide the same level of social protection as in France. And that makes a big difference for Indonesians and Nigerians! But a company still needs to have the economic means to offer this. Today, it is the companies that create value that can afford these types of employment conditions. With the crisis that erupted mid-2020 we will see how many groups still have the resources to implement these voluntary policies.
In the long term, we can imagine that this relationship may be reversed and that it will no longer be possible to create value without first being virtuous, as the majority of consumers, human talent and investors will no longer want to deal with companies that do not respect the environment and are not aware of their social responsibilities. It is no secret, though, that many new editions of the Vernimmen will be published before this becomes a reality.
SUMMARY
QUESTIONS
EXERCISE
ANSWERS
BIBLIOGRAPHY
NOTES
Chapter 27. MEASURING VALUE CREATION
Chapter 27
MEASURING VALUE CREATION
Separating the wheat from the chaff
Creating value has become such an important issue in finance that a host of indicators have been developed to measure it. They come under a confusing array of acronyms – TSR, MVA, EVA, CFROI, ROCE, WACC – but most of these will probably be winnowed out in the years to come. Ultimately, they should be reduced to those few that best mirror and address the recent developments in cash flow statements.
The current profusion of indicators has its advantages, as normally we expect only the most reliable to survive. However, in practice some companies use the lack of clear guidelines and standards to choose indicators that best serve their interests at a given time, even if this involves the laborious task of changing indicators on a routine basis.
The chart below should help you find your way through the maze of indicators. It plots value creation measures according to three criteria: ease of manipulation, sensitivity to financial markets and category (accounting, economic or stock market indicators).

Section 27.1 OVERVIEW OF THE DIFFERENT CRITERIA
Value creation indicators fall into four categories:
- Accounting indicators. Until the mid-1980s, companies mainly communicated their net profit/loss or earnings per share (EPS). Regrettably, this is a key accounting parameter that is also very easy to manipulate. This practice of massaging EPS is called “window dressing”, or improving the presentation of the accounts by adjusting exceptional items, provisions, etc. The growing emphasis on operating profit or EBITDA represents an improvement because it considerably reduces the impact of exceptional items and non-cash expenses. It is regrettable that the IASB has refused to define EBITDA, which leaves companies free to choose their own definition, whereas for us there is only one: the difference between all operating revenues and expenses that sooner or later results in a cash inflow or outflow; and which has eliminated exceptional items that are now included in the various expense items.
The second-generation accounting indicators appeared as investors began to reason in terms of profitability, i.e. efficiency, by comparing return with the equity used. One such ratio is called return on equity (ROE). However, it is possible to leverage this value as well, since a company can boost its ROE by skilfully raising its debt level. Even though ROE might look more attractive, no “real” value has been created since the increased profitability is cancelled out by higher risk not reflected in accounting data.
Since the return on capital employed (ROCE) indicator avoids this bias, it has tended to become the main measure of economic performance. Only in a few sectors of activity is it meaningless to use ROCE (essentially in banking or insurance, where ROE is widely used).
These rates mainly fall into the field of accounting, rather than finance.
While NPV and other economic indicators represent valuable tools for strategic analysis and a good basis for estimating the market value of companies, they are based on projections that are frequently difficult to assess. Unfortunately, the cash flow for one single year is easy to manipulate and meaningless. Indeed, it is not intuitively interpretable. At the same time, we know that the major drivers of cash flows are the growth of earnings and revenues of the company and ROCE. By focusing attention on ROCE, there is a better intuitive grasp of how the company is performing. It is then easier to assess the firm’s growth, both over time and relative to its industry.
- Accounting/financial indicators emerged with the realisation that profitability per se cannot fully measure value because it does not factor in risks. To measure value, returns must also be compared with the cost of capital employed. Using the cost of financing a company, called the weighted average cost of capital, or WACC,1 it is possible to assess whether value has been created (i.e. when return on capital employed is higher than the cost of capital employed) or destroyed (i.e. when return on capital employed is lower than the cost of capital employed).
But companies can also go one step further by applying the calculation to capital employed at the beginning of the year in order to measure the value created over the period. The difference can then be expressed in currency units rather than as a percentage. This popular measure of value creation has been most notably developed in the EVA, or economic value added, model. It is also known as economic profit.
These indicators are mainly used for decentralised management control and for the calculation of variable compensation, often linked directly or indirectly to economic profit.
- Financial indicators. Yet the best of all indicators is undoubtedly net present value (NPV, see Chapter 16), which provides the exact measure of value created. It has been repeatedly demonstrated that intrinsic value creation is the principal driver of companies’ market value. But NPV has one drawback because it must be computed over several periods. For the external analyst who does not have access to all the necessary information, the NPV criterion becomes difficult to handle. The quick and easy solution is to use the above-mentioned ratios. It is important to remember that while the other ratios are simpler to use, they are also less precise and may prove misleading when not used with care.
They are mostly used for investment choices and valuation.
- Market indicators: market value added (MVA) and total shareholder return (TSR) are highly sensitive to the stock market. MVA represents the difference on the one hand between the value of equity and net debt, and on the other the book value of capital employed. It is expressed in currency units. TSR is expressed as a percentage and corresponds to the addition of the dividend yield on the share (dividends/value of the share) and the capital gains rate (capital gains during the period divided by the initial share value). It is the return earned by a shareholder who bought the share at the beginning of a period, earned dividends and then sold the share at the end of the period.
A major weakness with these two measures is that they may show destruction in value because of declining investor expectations about future profits, even though the company’s return on capital employed is higher than its cost of capital. This is the case for Bic, which has seen its share price drop since 2015, despite having an average ROCE above 12% every year since then, for a cost of capital of roughly 7%. Conversely, in a bull market, a company with mediocre economic performance may have flattering TSR and MVA. In the long term, these highs and lows are smoothed out and TSR and MVA would eventually reflect the company’s modest performance. Yet in the meantime, there may be some major divergences between these indicators and company performance.
TSR is sometimes used as an index for variable compensations. MVA is rarely used.
A clear distinction must be made between economic indicators and measures of stock market value creation (TSR and MVA). The former measure the past year’s performance, while the latter tend to reflect anticipation of future value creation. The measures of stock market value creation take into account the share price, which reflects this anticipation. Yet the different measures of economic performance and stock market value are complementary, rather than contradictory.
Section 27.2 NPV, THE ONLY RELIABLE CRITERION
It should now be clear that the concept of value corresponds perfectly to the measure of NPV. Financial management consists of constantly measuring the net present value of an investment, project, company or source of financing. Obviously, one should only allocate resources if the net present value is positive; in other words, if the market value is lower than the present value. Net present value reflects how allocation of the company’s resources has led to the creation or destruction of value. On the one hand, there is a constant search for anticipated financial flows – while keeping in mind the uncertainty of these forecasts. On the other hand, it is necessary to consider the rate of return (k) required by the investors and shareholders providing the funds.
The value created is thus equal to the difference between the capital employed and its book value. Book value is the amount of funds invested in the company’s operations.
The creation of value reflects investors’ expectations. Typically, this means that, over a certain period, the company will enjoy a rent with a present value allowing its capital employed to be worth more than its book value!
While NPV is widely used within companies to make investment choices (Chapter 28), it is hardly ever used by the company in its external communication on its value creation.
If it were to do so, it would be obliged to give precise details of its business plan and future cash flows to the public. No company is prepared to do so in order not to give confidential information to its competitors, nor to give the impression of making performance commitments that it might not keep because it is dependent on economic and financial conditions that are beyond its control.
Hence the development of value creation indicators, which are much less indiscreet, but also often much less effective and much easier to manipulate!
Section 27.3 FINANCIAL/ACCOUNTING CRITERIA
1/ ECONOMIC PROFIT OR EVA
Economic profit is less ambitious than net present value. It only seeks to measure the wealth created by the company in each financial year. EVA does not just factor in the cost of debt, such as in calculating net profit, but also accounts for the cost of equity.
Economic profit or EVA first measures the excess of ROCE over the weighted average cost of capital. Then, to determine the value created during the period, the ratio is multiplied by the book value of thecapital employed at the start of the reporting period. Thus, a company that had an opening book value of capital employed of 100 and an after-tax return on capital employed of 12% with a WACC of only 10% will have earned 2% more than the required rate. It will have created a value of 2 on funds of 100 during the period.
Economic profit is related to net present value, because NPV is the sum of the economic profits discounted at the weighted average cost of capital:

The table shows EVA for some European firms.
| Company | 2020 EVA (€m) | Company | 2020 EVA (€m) |
|---|---|---|---|
| Roche | 12,800 | Adidas | (26) |
| Nestlé | 6,912 | Bonduelle | (39) |
| L’Oréal | 2,831 | E.ON | (54) |
| AstraZeneca | 1,164 | Heidelberg Cement | (102) |
| Peugeot | 576 | Michelin | (312) |
| Carrefour | 278 | BASF | (759) |
| RTL | 245 | Shell | (974) |
| Carlsberg | 169 | LafargeHolcim | (1,222) |
| Proximus | 102 | Telecom Italia | (1,913) |
| Criteo | 46 | ArcelorMittal | (2,485) |
| BIC | 14 | ENI | (3,557) |
| Heineken | 0 | Deutsche Telekom | (3,784) |
Source: Data from Exane BNP Paribas, Factset
To calculate EVA, it is necessary to switch from an accounting to an economic reading of the company. This is done by restating certain items of capital employed as follows:
- The exceptional losses of previous years must be restated and added to capital employed insofar as they artificially reduce the company’s capital.
- The goodwill recorded in the balance sheet must be taken as gross, i.e. corrected for cumulative amortisation or impairment, the badwill must be deducted from equity and assets.
- Other major restatements are for deferred tax liabilities and for depreciation (so as to be consistent with capital employed obtained through previously mentioned restatements).
Of course, the profit and loss account (operating profit/loss and taxes) must be restated to ensure consistency with the capital employed calculated previously.
The firms that develop economic profit tools for companies generally have a long list of accounting adjustments that attest to their expertise. Such accounting expertise typically represents a barrier to entry for others seeking to perform the same analyses.
EVA’s novelty also lies in its scope of application, since it enables a company to measure performance at all levels by applying an individual required rate of return to various units. It is a decentralised financial management tool.
A firm may be tempted to maximise short-term EVA, which may be detrimental to future EVAs (underinvestment, artificial reduction of working capital). In general, it is very complex to pick annual criteria that will make it possible to measure value creation for a firm properly. Only the NPV of future cash flows allows us to take into account the long-term capacity to create value.
2/ CASH FLOW RETURN ON INVESTMENT
The original version of cash flow return on investment (CFROI) corresponds to the average of the internal rates of return on the company’s existing investments. It measures the IRR earned by a firm’s existing projects.
CFROI is the internal rate of return that equals the company’s gross capital employed, i.e. before depreciation and adjusted for inflation, and the series of after-tax EBITDA computed over the lifetime of existing fixed assets (estimated by dividing the gross value of fixed assets by the depreciation). CFROI is then compared with the weighted average cost of capital. If CFROI is higher than WACC, then the company is creating value; if it is lower, then the firm is destroying value.
As with EVA, computing CFROI requires a number of restatements, which seem to exist mainly to convince their users to hire the founder of the concept (Holt) to implement it. It is sometimes used in a very simplified manner, which makes it very close to a mere accounting criterion (see Section 27.5).
Section 27.4 MARKET CRITERIA
1/ CREATING STOCK MARKET VALUE (MARKET VALUE ADDED)
For listed companies, market value added (MVA) is equal to:
In most cases, if no other information is available, we assume that net debt corresponds to its book value. Thus, the equation becomes simpler:
So, market value added is frequently considered to be the difference between market capitalisation and the book value of equity. This is the equivalent of the price-to-book ratio (PBR) discussed in Chapter 22.2 The table shows MVA for some large listed European companies.
| Company | 2020 MVA (€m) | Company | 2020 MVA (€m) |
|---|---|---|---|
| Adidas | 53,250 | NRJ | (170) |
| ABB | 36,716 | Peugeot | (533) |
| Sanofi | 36,080 | Heidelberg Cement | (1,124) |
| Deutsche Telekom | 35,286 | Stellantis | (5,268) |
| Ericsson | 23,974 | ENI | (6,594) |
| Cap Gemini | 15,299 | Natixis | (7,845) |
| Total | 8,900 | Orange | (8,502) |
| Michelin | 6,084 | ArcelorMittal | (11,992) |
| Telefonica | 6,056 | Renault | (14,197) |
| Nokia | 5,258 | Vodafone | (17,735) |
| Saint Gobain | 2,084 | NatWest | (20,146) |
| M6 | 616 | Porsche | (27,309) |
| Bonduelle | (12) | Crédit Agricole | (28,315) |
Source: Data from Exane BNP Paribas, Factset.
It is not complex to demonstrate the relationship between market value added and intrinsic value creation in equilibrium markets, since:

Economic profit being equal to capital employed × (ROCE − WACC). This is also equivalent to:
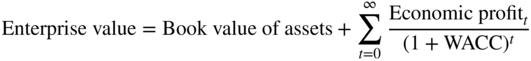
However, those who do not believe in market efficiency contend that MVA is flawed because it is based on market values that are often volatile and out of the management’s control. Yet this volatility is an inescapable fact for all, as that is how the markets function.
2/ TOTAL SHAREHOLDER RETURN (TSR)
TSR is the return received by the shareholder who bought the share at the beginning of a period, earned dividends (which are generally assumed to have been reinvested in new shares) and values their portfolio with the last share price at the end of the period. In other words, TSR equals (share appreciation + dividends)/price at the beginning of the period.
In order for it to be meaningful, the TSR ratio is calculated on a yearly basis over a fairly long period of, say, 5–10 years. This smooths out the impact of erratic market movements, e.g. the tech, media and telecom stock bubble of 2000, the 2007–2010 or the Covid-19 crisis.
By way of illustration, here is the TSR of a few major groups over the last 10 years.
| Company | TSR over 10 years | Company | TSR over 10 years |
|---|---|---|---|
| Apple | 30.7% | Rio Tinto | 7.6% |
| L’Oréal | 16.2% | Vinci | 6.3% |
| Allianz | 11.2% | Total | 4.6% |
| Nestlé | 10.5% | ICBC | 3.7% |
| Infosys | 10.2% | IBM | 1.7% |
| Enel | 10.1% | General Electric | –1.3% |
| Sanofi | 9.4% | Vale | –1.6% |
| Toyota | 9.3% | Nokia | –1.8% |
| Siemens | 9.3% | Santander | –5.7% |
| Coca-Cola | 8.3% | Cathay Pacific | –6.5% |
Source: Data from DQYDJ and ycharts
Section 27.5 ACCOUNTING CRITERIA
Certain accounting indicators, like net profit, shareholders’ equity and cash flow from operations, are more representative of a firm’s financial strength. As such they are flawed to measure value creation.
Earnings per share and the accounting rates of return (ROCE, ROE), whilst systematically used as analytical criteria for all financial decisions, even at the board level, are not without faults as they largely ignore risk due to their accounting origin.
It is inappropriate to believe that by artificially boosting them you have created value. Nor is it correct to assume that there is a constant and automatic link between improving these criteria and creating value. In order to maximise value, it is simply not enough to maximise these ratios, even if they are linked by a coefficient to value or the required rate of return.
1/ EARNINGS PER SHARE
Notwithstanding the comments just made about earnings per share, many financial managers continue to favour using it, especially with regard to financial communication. Despite its limitations, it is still the most widespread multiple because it is directly connected to the share price via the price/earnings ratio. EPS’s popularity is rooted in three misconceptions:
- the belief that earnings per share factors in the cost of equity and, therefore, the cost of risk;
- the belief that accounting data influence the value of the company. Changing accounting methods (for inventories, depreciation, goodwill, etc.) will not modify the company’s value, even if it does change earnings per share; and
- the belief that any financial decision that lifts EPS will change value as well. This would imply that the P/E ratio3 remains the same before and after the financial decision, which is frequently not the case. Thus, value is not a direct multiple of earnings per share, because the decision may affect investors’ assessment of the company’s risks and growth potential.
Consider Company A, which, based upon its risks and growth and profitability prospects, has a P/E ratio of 20. Its net profit is 50. Company B has equity of 450 with net profit of 30, giving it a P/E of 15. Company A decides to acquire a controlling interest in Company B, paying a premium of 33% on B’s value, i.e. a total of 600. Company A finances the acquisition entirely by taking on debt at an after-tax cost of 3%. Both Companies A and B are fairly valued. There are no industrial or commercial synergies that could increase the new group’s earnings, and no goodwill.
Company A’s net profit is thus:
Since A financed its acquisition of B entirely through debt, it still has the same number of shares. The increase in earnings per share, also called earnings per share accretion, is therefore equal to that in net profit; that is, +24%. This certainly seems like an extraordinary result!
But has A really created value by buying B?
The answer is no, since there are no synergies to speak of between A and B. Keep in mind that A paid 33% more than B’s equilibrium price. In fact, Company A has destroyed value in proportion to this premium, i.e. 150, because it cannot be offset by synergies.
In fact, the explanation for the – apparent – paradox of a 24% rise in earnings per share matched by a destruction of value is that the buyer’s EPS has increased, because the P/E ratio of the company bought by means of debt is higher than the after-tax cost of the debt. Here, B has a P/E ratio of 20 given the 33% premium paid by A on the acquisition. The inverse of 20 (5%) is much higher than the 3% after-tax cost of the debt for A.
Consider now Company C, which has equity of 1,400 with net profit of 140, i.e. a P/E of 10. It merges with Company D, which has the same risk exposure, equity of 990 and a P/E of 18 (net profit of 55), with no control premium. Thanks to very strong industrial synergies, C is able to boost D’s net profit by 50%. Without doubt, value has been created. And yet, it is not difficult to prove (see Exercise 1) that C’s EPS dropped 7% after the merger. This is a mechanical effect due simply to the fact that D’s P/E of 18 is higher than C’s P/E of 10, because D has better earnings prospects than C.
So, what was the net result of Company C’s acquisition of Company D? The question is not whether Company C’s EPS has been enhanced or diluted, but whether it paid too much for D. In fact, it did not, since there was no control premium paid and industrial synergies were created. After the operation, C’s share will trade at a higher P/E ratio, as it should enjoy greater earnings growth thanks to the contribution from D’s higher-growth businesses. In the end, C’s higher P/E ratio should more than compensate for C’s diluted EPS, lifting the share price. This is only logical considering that the industrial synergies created value.
If these three conditions are met, we can assume that EPS growth reflects the creation of value, and EPS dilution the destruction of value.
If just one of these conditions is lacking, then there is no way to infer that any increase in EPS reflects the creation of value, nor that a decrease is a destruction of value. In our example of a combination between A and B financed by debt, although A’s EPS rose 24%, its risk increased sharply. Its position is no longer directly comparable with that before the acquisition of B.
Similarly, C’s post-merger EPS cannot be compared with its EPS prior to the merger. While the merger did not change its financial structure, C’s growth rate after the merger with D is different from what it was beforehand.
2/ EARNINGS PER SHARE ADJUSTED FOR THE COST OF CARBON EMISSIONS
Used for the first time by Danone in February 2020, this adjusted EPS makes the cost of the carbon footprint visible in financial performance. It measures the accounting enrichment of the shareholder, in relation to one share, once the cost of the carbon credits that the company would have to acquire to offset its greenhouse gas emissions is taken into account. Danone has estimated them on the basis of a cost of €35 per tonne of carbon.
The cost of the externalities that the company causes to the community is thus integrated into the company’s financial performance, thus finally linking the two worlds of finance and non-finance.
At a constant cost of carbon credits, this EPS will grow faster than traditional EPS for companies that reduce their carbon footprint, which should enable investors to value them better. It is simply calculated from the net profit (group share) used to calculate EPS, from which the estimated greenhouse gas emissions multiplied by the price per tonne of carbon is subtracted, before dividing the balance by the number of diluted shares.
Once this calculation has been made, there is no reason why the other value creation indicators presented in this chapter cannot be adjusted in the same way.
It may be that the current EPS adjusted for carbon costs will not ultimately be the criterion retained by the market or the most relevant, but we have to start somewhere … and someone has to start.
3/ ACCOUNTING RATES OF RETURN
Accounting rates of return comprise:
- return on equity (ROE);
- return on capital employed (ROCE), which was described in Chapter 13; and
- cash flow return on investment (CFROI), the simplified version of which compares EBITDA with gross capital employed, i.e. before amortisation and depreciation of fixed assets.

This ratio is used particularly in business sectors wherein charges to depreciation do not necessarily reflect the normal deterioration of fixed assets, e.g. in the hotel business.
The main drawback of accounting rates of return on equity or capital employed is precisely that they are accounting measures. As shall be demonstrated below, these have their dangers.
Consider5 Company X, which produces a single product and generates a return of 20% on capital employed amounting to 100. X operates in a highly profitable sector and is considering external growth opportunities. Should it expect the present 20% rate of return to be generated on other possible projects? If it does, X will never invest because it is unlikely that any other investments will meet these criteria.
How can this problem be rationally approached? The company generates an accounting return of 20%. Suppose its shareholders and investors require a 10% return. Its market value is thus 20 / 10%, or 200.
The proposed investment amounts to 100 and generates a return of 15% on identical risks. The required rate of return is constant at 10%. We see that:
This yields an enterprise value of 35 / 10% = 350 (+150), with a return on capital employed of 35 / 200 = 17.5%.
The value of the capital employed has increased by more than the amount invested (150 vs. 100), because the profitability of Company X’s investment (15%) is higher than the rate required by its shareholders and investors (10%). Value has been created, and X was right to invest. And yet, the return on capital employed fell by 20% to 17.5%, demonstrating that this criterion is not relevant.
The inverse example is Company Y, which has a return of 5% on capital employed of 100. Assuming the shareholders and investors require a 10% return as well, the value of Y’s capital employed is 5 / 10% = 50.
The proposed investment amounts to 25 and yields a return of 8%. Since we have the same 10% required return, we get:
This results in capital employed being valued at 7 / 10% = 70 (+20), with a return of 7 / 125 = 5.6%.
The value of Y’s capital employed has indeed increased by 20, but this is still less than the increase of 25 in capital invested. Value has been destroyed. The return on the investment is just 8%, whereas the required rate is 10%. The company has lost money and should not have made the investment. And yet the return on capital employed rose from 5% to 5.6%.
Similarly following an acquisition financed with a share issue, one could demonstrate that ROE increases when the target company’s reverse 1 / (P/E) is higher than the buyer’s current ROE.
Setting aside all these accounting concepts, to focus on financial concepts, such as requested rates of return (k).
Unfortunately, some corporate managers continue to view decision-making in terms of the impact on accounting measures (EPS and accounting profitability), even though it has just been demonstrated that these criteria have little to say about the creation of value. True, accounting systems are a company’s main source of information. However, financial managers need to focus first and foremost on how financial decisions affect value.
Let’s discuss synergy effects before mentioning EPS accretion!
Section 27.6 SYNTHESIS
As long as performance measures and their implementation remain so diversified, it is vital to have a good understanding of their respective flaws. By choosing one or another measure, companies can present their results in a more or less flattering light. Financial managers typically choose those measures that will demonstrate the creation, rather than the destruction, of value.
| Financial criteria | Financial/accounting criteria | Accounting criteria | Market criteria | |||||
|---|---|---|---|---|---|---|---|---|
| Ratio | Net present value | Economic profit | Cash flow return on investment | Earnings per share | Accounting rates of return | Market value added | Total shareholder return | |
| Acronym | NPV | EVA | CFROI | EPS | ROE, ROCE | MVA | TSR | |
| Strengths | The best criterion | Simple indicator leading to the concept of weighted average cost of capital | Not restricted to just one year | Historical data; simple | Simple concepts | Astoundingly simple; reflects total rather than annual value created | Represents shareholder return in the medium to long term | |
| Weaknesses | Difficult to calculate for an external analyst | Restricted to one year; difficult to evaluate changes over a period of time | Complex calculations | Does not factor in risks; easily manipulated; does not factor in the cost of equity | Accounting measures, thus do not factor in risks; restricted to one year; to be significant, must be compared with the required rate of return | Subject to market volatility; difficult to apply to unlisted companies | Calculated over too short a period; subject to market volatility | |
SUMMARY
QUESTIONS
EXERCISES
ANSWERS
BIBLIOGRAPHY
NOTES
- 1 See Chapter 29. Cost of capital is the weighted average cost of financial resources (equity and debt) available for the firm. It is therefore the minimum return that the company shall provide in order to satisfy the fund providers and hence create value.
- 2 The market-to-capital ratio is a variation of MVA expressed as a ratio rather than a unit amount, because it is obtained by dividing the market capitalisation of debt and equity by the amount of capital invested.
- 3 The P/E ratio is equal to price/earnings per share. It measures the relative expense of a share.
- 4 Before goodwill accounting.
- 5 To simplify the discount calculation, we assume that the planned investments will generate a return to infinity.
Chapter 28. INVESTMENT CRITERIA
Chapter 28
INVESTMENT CRITERIA
Back to flows and financial analysis
The “mathematics” we studied in Chapters 16 and 17, dealing with present value and internal rate of return, can also be applied to investment decisions and financial securities. These theories will not be covered again in detail, since the only real novelty is of a semantic nature. In the sections on financial securities, we calculated the yield to maturity. The same approach holds for analysing industrial investments, whereby we calculate a rate that takes the present value to zero. This is called the internal rate of return (IRR). Internal rate of return and yield to maturity are thus the same.
This chapter will discuss:
- the cash flows to be factored into investment decisions, which are called incremental cash flows; and
- other investment criteria, which are less relevant than NPV and IRR and have proven disappointing in the past. As financial managers, you should nevertheless be aware of them, even if they are more pertinent to accounting work than financial management: payback period, accounting rate of return, profitability indicator.
Section 28.1 THE PREDOMINANCE OF NPV AND THE IMPORTANCE OF IRR
Each investment has a net present value (NPV), which is equal to the amount of value created. Remember that the net present value of an investment is the value of the positive and negative cash flows arising from an investment, discounted at the rate of return required by the market. The rate of return is based upon the investment’s risk.
From a financial standpoint, and if forecasts are correct, an investment with positive NPV is worth making since it will create value. Conversely, an investment with negative NPV should be avoided as it will destroy value.
Sometimes investments with negative NPV are made for strategic reasons, such as to protect a position in the industry sector or to open up new markets with strong, yet hard-to-quantify, growth potential. It must be kept in mind that if the NPV is really negative, it will certainly lead to the destruction of value. Sooner or later, projects with negative NPV have to be offset by other investments with positive NPV that create value. Without doing so, the company will be headed for ruin.
The internal rate of return (IRR) is simply the rate of return on an investment. Given an investment’s degree of risk, it is financially worthwhile if the IRR is higher than the required return. On the other hand, if the IRR is lower than the risk-based required rate of return, the investment will serve no financial purpose.
Net present value (NPV) measures the value created by the investment and is the best criterion for selecting or rejecting an investment, whether it is industrial or financial. When it is simply a matter of deciding whether or not to make an investment, NPV and IRR produce the same outcome. However, if the choice is between two mutually exclusive investments, net present value is more reliable than the internal rate of return.
From a conceptual and methodological point of view, NPV is a better criterion as it takes into account risk (payback ratio does not), the whole stream of cash flows (idem) and assumes that intermediate cash flows are reinvested at the cost of capital, which is more realistic than IRR (which implicitly assumes reinvestment at the IRR, which may be above the cost of capital).
Actual computation of NPV is not always well applied. Indeed, some managers discount cash flows using the cost of capital of the group and not at a rate that reflects the market risk of the specific project. It should be kept in mind that a very risky project will increase the overall risk of the firm and thus should be discounted at a higher rate (and vice versa). We will highlight this point in the next chapter.
Graham and Harvey (May 2001) conducted a broad survey of corporate and financial managers to determine which tools and criteria they use when making financial decisions. They asked them to indicate how frequently they used several capital budgeting methods. The findings showed that net present value and internal rate of return carry the greatest weight, and justifiably so. Some 75% of financial managers systematically value investments according to these two criteria. This proportion increases over time demonstrating that pedagogy in finance is not useless.
Interestingly, large firms apply these criteria more often than small and medium-sized companies, and MBA graduates use them systematically while older managers tend to rely on the payback ratio.
Conclusions are slightly different for small and medium companies for which (according to a study by Danielson and Scott) intuition comes first (26%), then payback ratio (19%), ROCE (14%) and NPV (12%).
Section 28.2 THE MAIN LINES OF REASONING
All investment decisions must comply with the following six principles:
- consider cash flows rather than accounting data;
- reason in terms of incremental cash flows, considering only those associated with the project;
- reason in terms of opportunity;
- disregard the type of financing;
- consider taxation; and
- above all, be consistent.
1/ REASON IN TERMS OF CASH FLOWS
We have already seen that the return on an investment is assessed in terms of the resulting cash flows. Indeed, only cash flows can be invested and earn interest or be used to repay a debt and stop the payment of its interests. One must therefore analyse the negative and positive cash flows, and not the accounting income and expenses. These accounting measures are irrelevant because they do not take into account working capital generated by the investment and include depreciation, which is a non-cash item.
We stress the fact that in finance, an amount costs only when it is disbursed and earns only when it is received, regardless of the accounting treatment applied to it.
2/ REASON IN TERMS OF INCREMENTAL FLOWS
When considering an investment, one must take into account the flows it generates, all the flows derived from the investment, and nothing else but these flows. It is crucial to assess all the consequences of an investment upon a company’s cash position. Some of these are self-evident and easy to measure, and others are less so.
A movie theatre group plans to launch a new complex, and substantial costs have already been incurred in its design. Should these be included in the investment’s cash flows? The answer is no, since the costs have already been incurred regardless of whether or not the complex is actually built. These are sunk costs. Therefore, they should not be considered part of the investment expenditure.
It would be absurd to carry out an investment simply because the preparations were costly and one hopes to recoup funds that, in any case, have already been spent. The only valid reason for pursuing an investment is that it is likely to create value.
Now, if the personnel department has to administer an additional 20 employees hired for the new complex (e.g. 5% of its total workforce), should 5% of the department’s costs be allocated to the new project? Again, the answer is no. With or without the new complex, the personnel department is part of overhead costs. As a general rule, structural costs cannot be attributed, even in part, to an investment because they are independent of it. Structural expenses would only be affected if the planned investment generates additional costs – which in our example is recruitment expenses.
However, design and overheads will be priced (to the extent possible given the competitive environment) into the ticket charged for entry to the new complex. Finance here differs quite markedly from management control.
A perfume company is about to launch a new product line that may cut sales of its existing perfumes by half. Should this decline be factored into the calculation of the investment’s return? Yes, because the new product line will prompt a shift in consumer behaviour: the decline in cash flow from the older perfume stems directly from the introduction of this new product.
Nevertheless, we can mention that in certain very specific sectors with very low marginal costs, this reasoning may lead to overinvestment, creating overcapacity and therefore price wars.
3/ REASON IN TERMS OF OPPORTUNITY
For financial managers, an asset’s value is its market value, which is the price at which it can be bought (investment decision) or sold (divestment decision). From this standpoint, its book or historic value is of no interest whatsoever, except for tax purposes (taxes payable on book capital gains, tax credit on capital losses, etc.).
For example, if a project is carried out on company land that was previously unused, the land’s after-tax resale value must be considered when valuing the investment. After all, in principle, the company can choose between selling the land and booking the after-tax sales price, or using the land for the new project. Note that the book value of the land does not enter into this line of reasoning.
The opportunity principle boils down to some very simple rules:
- if a company decides to hold on to a business, this implies that it should be prepared to buy that business (if it did not already own it) in identical operating circumstances; and
- if a company decides to hold on to a financial security that is trading at a given price, this security is identical to one that it should be prepared to buy (if it did not already own it) at the same price.
Financial managers are, in effect, “asset dealers”. They must introduce this approach within their company, even if it means standing up to other managers who view their respective business operations as essential and viable. Only by systematically confronting these two viewpoints can a company balance its decision-making and management processes.
Theoretically, a financial manager does not view any activity as essential, regardless of whether it is one of the company’s core businesses or a potential new venture. The CFO must constantly be prepared to question each activity and reason in terms of:
- buying and selling assets; and
- entering or withdrawing from an economic sector of activity.
The concept of necessity should be interpreted as regards the strategy of the firm, the investment is then a tool for achieving this strategy; a necessary tool, hence highly profitable.
4/ DISREGARD THE TYPE OF FINANCING
When comparing an investment’s return with its cost of financing, the two items must be considered separately.
In practice, since the discount rate corresponds to the required rate of return necessary to cover the total cost of financing the investment, interest expense, repayments or dividends should not be included in the flows. Only operating and investment flows are taken into account, but never financing flows. This is the same distinction that was made in Chapter 2. Failure to do so would skew the project’s net present value. This would also overstate its IRR, since the impact of financing would be included twice:
- first, within the weighted average cost of capital for this investment, which is its cost of financing; and
- second, at the cash flow level.
To demonstrate this, consider, for example, an investment with the following flows:
| Year | 0 | 1 | 2 | 3 |
|---|---|---|---|---|
| Investment flows | −100 | 15 | 15 | 115 |
The NPV of this investment is 7.2 (if cash flows are discounted at 12%) and its IRR is 15%. Now, assume that 20% of the investment was financed by debt at an annual after-tax cost of 6%. Then it is possible to deduct the debt flows from the investment flows and calculate its NPV and IRR:
| Year | 0 | 1 | 2 | 3 |
|---|---|---|---|---|
| Investment flows | −100 | 15.0 | 15.0 | 115.0 |
| Debt financing flows | 20 | −1.2 | −1.2 | −21.2 |
| Net flows to equity | −80 | 13.8 | 13.8 | 93.8 |
With a rate of 12%, the NPV is 10.1 and the IRR is 17.2%. Now, if 50% of the investment were financed by debt, the NPV would rise to 14.4 and the IRR to 24%. At 80% debt financing, NPV works out to 18.7 and the IRR to 51%.
This demonstrates that by taking on various degrees of debt, it is possible to manipulate the NPV and IRR. This is the same as using the financial leverage that was discussed in Chapter 12. However, this is a slippery slope. It can lead unwary companies to invest in projects whose low industrial profitability is offset by high debt, which in fact increases the risk considerably.
When debt increases, so does the required return on equity as the risk increases for shareholders, as we have seen in Chapter 12. It would be incorrect to continue valuing the previous NPV at a constant discount rate of 12%. The discount rate has to be raised in conjunction with the level of debt. This corrects our reasoning and NPV remains constant. The IRR is now higher, but the minimum required return has risen as well to reflect the greater degree of risk of an investment financed by borrowings.
It would be absurd to believe that one can undertake an investment because it generates an IRR of 10% whereas the corresponding debt can be financed at a rate of 7%. In fact, the debt is only available because the company has equity that acts as collateral for creditors. Equity has to be remunerated, and this is not reflected in the 7% interest on the debt. No company can be fully financed by debt, and it is therefore impossible to establish a direct comparison between the cost of debt and the project’s return.
5/ CONSIDER TAXATION
Clearly, taxation is an issue because corporate executives endeavour to maximise their after-tax flows; it goes without saying that this is done while respecting fiscal regulations. Consider that:
- additional depreciation generates tax savings that must be factored into the equation;
- the cash flows generated by the investment give rise to taxes, which must be included as well; and
- certain tax shields offer tax credits, carbon credits, rebates, subsidies, allowances and other advantages for carrying out investment projects.
In practice, it is better to value a project using after-tax cash flows and an after-tax discount rate in order to factor in the various tax benefits from an investment. Therefore, the return required by investors and creditors is calculated after tax.
In cases where cash flows are discounted before tax, it is important to ascertain that all flows and components of weighted average cost of capital are considered before taxes as well.
6/ BE CONSISTENT!
The best advice we can give to our readers is to always be consistent. If the basis of valuation is constant euro values – that is, excluding inflation – be sure that the discount rate excludes inflation as well. We recommend using current euro values, because the discount rate already includes the market’s inflation expectations.
If it is a pre-tax valuation, make sure the discount rate reflects the pre-tax required rate of return. We recommend using after-tax valuations because a world without taxes only exists in textbooks!
And if flows are denominated in a given currency, the discount rate must correspond to the interest rate in that currency as well.
7/ AND WHAT ABOUT ENERGY TRANSITION?
Should the urgency of energy transition not lead to the adoption of new investment selection criteria in order to facilitate it? We do not think so. Current criteria can quite easily account for incentives favouring energy transition, be it in terms of flows (subsidies, carbon credits, etc.) or the discount rate, as we will see in Chapter 29.
This being said, a company is not forced to select, from among mutually exclusive investments, the one with the best NPV if it goes with a high carbon footprint. It should be noted that such a situation would reflect an unsatisfactory incentive/regulatory balance put in place by governments. The company may even decide to hold on to an investment with a negative NPV in order to help the planet. But in a competitive market, a firm cannot be virtuous on its own. It will find it difficult to behave in this way unless, on the other hand, it makes investments with a positive NPV to offset its virtuous behaviour.
Section 28.3 WHICH CASH FLOWS ARE RELEVANT?
In practice, three types of cash flow must be considered when assessing an investment: operating flows, investment flows and extraordinary flows. Financial managers try to plan both the amount of a cash flow and its timing. In other words, they draw up projections of the cash flows on the investment.
Where the investment has a limited life, it is possible to anticipate its cash flows over the entire period. But, in general, the duration of an investment is not predetermined, and one assumes that at some point in the future it will be either wound up or sold. This means that the financial manager has to forecast all cash flows over a given period with an explicit forecast period, and reason in terms of residual (or salvage) value beyond that horizon. The residual value reflects the flows extending beyond the explicit investment horizon, and on into infinity. Although the discounted residual value is frequently very low since it is very far off in time, it should not be neglected. Its book value is sometimes zero, but its economic value may be quite significant since accounting depreciation may differ from economic depreciation. If some of the assets may be sold off, one must also factor in any taxes on capital gains.
1/ OPERATING FLOWS
The investment’s contribution to total earnings before interest, taxes, depreciation and amortisation (EBITDA) must be calculated. It represents the difference between the additional income and expenses arising from the investment, excluding depreciation and amortisation.
Then, from EBITDA, the theoretical tax on the additional operating profit must be deducted. This tax is then calculated by multiplying the tax rate borne by the project with the differential on the operating profit, taking into account any tax-loss carryforwards which could be used.
It is essential to deduct changes in working capital from EBITDA. Unfortunately, many people tend to forget this. In most cases, working capital is just a matter of a time lag. It builds up gradually, grows with the business and is retrieved when the business is discontinued. A euro capitalised today in working capital can be retrieved in 10 years’ time, but it will not be worth the same. Money invested in working capital is not lost. It is simply capitalised until the investment is discontinued. However, this capitalisation carries a cost, which is reflected in the discounted amount.
2/ INVESTMENT FLOWS
Investment in fixed assets comprises investment in maintenance, production capacity and growth, whether in the form of tangible assets (machinery, land, buildings, etc.) or intangible assets (research and development, patents and licences, business capital, etc.) or financial assets (shares in subsidiaries) for external growth.
The investment must be assessed for each period, as the investment is not necessarily restricted to just one year, nor spread evenly over the period. Once again, remember that our approach is based on cash and not accounting data. The investment flows must be recognised when they are paid, not when the decisions to make them were incurred. And finally, do not forget to reason in terms of net investment; that is, after any disposals, investment subsidies and other tax credits.
3/ EXTRAORDINARY FLOWS
It may seem surprising to mention extraordinary items when projecting estimated cash flows. However, financial managers frequently know in advance that certain expenses that have not been booked under EBITDA (litigation, tax audits, etc.) will be disbursed in the near future. These expenses must all be included on an after-tax basis in the calculation of estimated free cash flow.
Extraordinary flows can usually be anticipated at the beginning of the period since they reflect known items. Beyond a two-year horizon, it is generally assumed that they will be zero.
This gives us the following cash flow table:
| Periods | 0 | 1 | … | n |
|---|---|---|---|---|
| Incremental EBITDA | + | + | + + | |
| − Incremental tax on operating profit | − | − | − | |
| − Change in incremental working capital | − − | − − | − | + + |
| − Investments | − − − | − | − | |
| + Divestments after tax | + | + | + + | |
| − Extraordinary marginal expenses | − | |||
| = Cash flow to be discounted = Free cash flows | − − | + | + | + + |
Section 28.4 OTHER INVESTMENT CRITERIA
1/ THE PAYBACK PERIOD
The payback period is the time necessary to recover the initial outlay on an investment. Where annual free cash flows are identical, the payback period is equal to:
For the following investment:
| Period | 0 | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| Cash flows | −2.1 | 0.8 | 0.8 | 0.8 | 0.8 | 0.8 |
the payback period is 2.1 / 0.8 = 2.6 years.
Where the annual flows are not identical, the cumulative cash flows are compared with the amount invested, as below:
| Period | 0 | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| Cash flows | −1 | 0.3 | 0.4 | 0.4 | 0.5 | 0.2 |
| Cumulative cash flows | 0.3 | 0.7 | 1.1 | 1.6 | 1.8 |
The cumulative flow is 0.7 for period 2 and 1.1 for period 3. The payback period is thus two to three years. A linear interpolation gives us a payback period of 2.75 years.
Once the payback period has been calculated, it is compared with a cut-off date determined by the financial manager. If the payback period is longer than the cut-off period, then the investment should be rejected. Clearly, when the perceived risk on the investment is high, the company will look for a very short payback period in order to get its money back before it is too late!
The payback ratio is used as an indicator of an investment’s risk and profitability. However, it can lead to the wrong decision, as shown in the example below of investments A and B.
| Flows in period 0 | Flows in period 1 | Flows in period 2 | Flows in period 3 | Recovery within | 20% NPV | |
|---|---|---|---|---|---|---|
| Investment A | −1,000 | 500 | 400 | 600 | 2 years and 2 months | 42 |
| Investment B | −1,000 | 500 | 500 | 100 | 2 years | −178 |
The payback rule would prompt us to choose investment B, even though investment A has positive NPV but B does not. The payback rule can be misleading because it does not take all flows into account.
Moreover, because it considers that a euro today is worth the same as a euro tomorrow, the payback rule does not factor in the time value of money. To remedy this, one sometimes calculates a discounted payback period representing the time needed for the project to have positive NPV. Returning to the example, with a 20% discount rate, it then becomes:
| Year | 0 | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| Cumulative present values | −2.1 | −1.43 | −0.88 | −0.41 | −0.03 | 0.29 |
The discounted payback period is now 4 years compared with 2.6 years before discounting. Discounted or not, the payback period is a risk indicator, since the shorter it is, the lower the risk of the investment. That said, it ignores the most fundamental aspect of risk: the uncertainty of estimating liquidity flows. Therefore, it is just an approximate indicator since it only measures liquidity.
However, the payback ratio is fully suited to productive investments that affect neither the company’s level of activity nor its strategy. Its very simplicity encourages employees to suggest productivity improvements that can be seen to be profitable without having to perform lengthy calculations. It only requires common sense. However, calculating flows in innovative sectors can be something of a shot in the dark.
It should be noted that some companies calculate the NPV of their potential investments over a limited period (5 years for example); cash flows beyond this period are considered too uncertain and are neglected. In such cases, the practice is equivalent to the discounted payback period.
2/ RETURN ON CAPITAL EMPLOYED
The return on capital employed (ROCE) represents the increase in after-tax operating profit generated by the investment over the year divided by the capital employed (sum of fixed assets and the working capital generated by the investment):
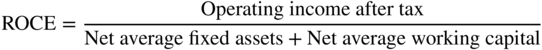
The average accounting return can also be calculated, which is the average of annual ROCEs over the life of the investment. The computation of ROCE takes into account the after-tax operating profit and capital employed (working capital plus the residual investment after depreciation).
By not taking into account when cash flows occur accounting rates of return generally overstate the rates of return (as high returns are more distant in time). Another drawback with accounting rates of return is that they maximise rates without considering the corresponding risk.
On the surface, it may seem that there is no connection between return on capital employed and the internal rate of return. The first discounts flows, while the second calculates book wealth. And yet, taken over a year, their outcomes are identical. An amount of 100 that increases to 110 a year later has an IRR of 100 = 110 / (1 + r), so r = 10%, and ROCE of 10 / 100, or 10%.
ROCE and IRR are equal over a given period of time. ROCE is therefore calculated by period, while IRR and NPV are computed for the entire life of the investment.
Although accounting rates of return should not be used as investment or financing criteria, they can be useful financial control tools.
Sooner or later, an IRR has to be translated into an accounting rate of return. If not, the investment has not generated the anticipated ex-post return and has not achieved its purpose. We strongly advise you to question any differences between IRR and ROCE, i.e. do profits arise unevenly over the period (starting out slowly or not at all and then gathering momentum), what is the amount of terminal value, are there calculation errors, is the hypothesis on the return of cash flows ploughed back in the business correct, etc.?
3/ CAPITAL RATIONING AND THE PRESENT VALUE INDEX
Sometimes there is a strict capital constraint imposed on the firm, and it is faced with more NPV-positive projects than it can afford. In order to determine which project(s) to pursue, some use the present value index (PVI). This is the present value of cash inflows divided by the present value of cash outflow:
By using the PVI, financial managers can rank the different projects and then select the investment with the highest PVI – that is, the project with the highest NPV relative to the present value of outflows. After making this selection, if the total amount of capital available has not been fully exhausted, the managers should then invest in the project with the second-highest PVI, and so on until no more capital remains to invest as long as PVI remains above 1.
Our reader will have noticed that just like the IRR, the profitability index does not take into account the size of the project, and two projects with the same IP can generate very different NPVs!
SUMMARY
QUESTIONS
EXERCISES
ANSWERS
BIBLIOGRAPHY
Chapter 29. THE COST OF CAPITAL
Mirror, mirror on the wall …
Determining the cost of capital, or weighted average cost of capital (WACC), is not a simple task, but it is one of the fundamentals of finance. The cost of capital has to be factored into investment decisions because it is the rate that is used for discounting cash flows for NPV or comparing with the IRR. Cost of capital is also used to determine enterprise value (see Chapter 31). Truly, its importance can hardly be understated.
But before reading on, it is imperative to understand the distinction between cost of capital, which is the weighted average cost of the capital contributed to the firm by its shareholders and its lenders, and cost of equity, which is just one component of the weighted average of the cost of capital. The reader should also take care not to rely excessively on spreadsheets without any prior reflection.
Section 29.1 THE COST OF CAPITAL AND THE RISK OF ASSETS
When markets are in equilibrium, any investor with a perfectly diversified portfolio holds a fraction of both the company’s equity and its debt. This is known as the CAPM, as was discussed in Chapter 19. In other words, each investor holds a share of the company’s operating assets, since this is equal to the sum of equity and net debt. Accordingly, each investor has some exposure to the risk arising from the company.
The rate of return required by investors thus depends on just one factor: the risk arising from the assets-in-place. This means that the cost of the company’s financial resources – its cost of capital – is none other than the rate of return required by investors, which is a function of the risk on capital employed.
The cost of capital is thus shaped by the economic characteristics of each sector of activity:
- The sensitivity to the economic environment: certain sectors structurally leverage changes in the economic climate. This is the case for transportation or civil works (high economic risk and hence cost of capital). Other sectors are less sensitive to downturns; this is the case for the agri-food industry.
- The cost structure (fixed vs. variable costs): the higher the fixed costs (in the cement or steel industries, for example), the more sensitive the firm is to the economic environment and the higher its cost of capital.
- The predictability of the activity: between a shopping mall operator benefiting from long-term rents and a fighter aircraft manufacturer, there is quite some difference in terms of predictability of revenues and cash flows. Their cost of capital will differ significantly: low for the shopping mall operator, high for the fighter aircraft manufacturer.
- The results growth rates: the higher the growth of future results, the higher the cost of capital. In such cases, the bulk of the enterprise value is due to cash flows which are distant in time and therefore quite sensitive to market fluctuations.
Modigliani and Miller (1958) and Miller (1977)1 were the first to state that the company’s cost of capital is not a function of its capital structure.
If the risk on capital employed is such that it requires a 12% rate of return, and if it is fully equity-financed, then shareholders will expect a minimum 12% return. On the other hand, if it is fully debt-financed, creditors will again require a 12% rate of return since they incur the same risk with the operating assets as the shareholders in the previous example. Lastly, suppose financing is equally divided between debt and equity. If the cost of debt is 6%, then shareholders will require an 18% return on equity to achieve a weighted average of 12%, i.e. the remuneration justified by the 12% risk for capital employed or the cost of capital.
Assume that, in a perfect market, the company changes its capital structure – for example, by buying back some of its equity via the issue of new debt. In this case, an investor with a perfectly diversified portfolio who holds 1% of the company’s equity and 1% of its debt and thus 1% of its capital employed will continue to hold 1% of capital employed, though now with a lower amount of equity because of the share buy-back and a higher percentage of net debt. The transaction is thus totally neutral for the investor. It will not affect the cost of capital, even if it is now divided between the cost of debt and the cost of equity, because the risk on capital employed remains unchanged.
As we have already discussed, the cost of capital is equal to the weighted average costs of net debt and of net equity. This will be examined in greater detail in the next section.
It is fundamental to understand this. We shall see that it is only for practical purposes that the cost of capital is reconstituted from the respective costs of net debt and equity.
Section 29.2 COMPUTING THE COST OF CAPITAL
The cost of capital can be calculated in three ways: directly, indirectly or via enterprise value.
1/ DIRECT CALCULATION VIA THE β OF ASSETS
Since a company’s liabilities merely provide a “screen” between the asset side of the company and the financial market, the rate of return required to satisfy investors is equal to the risk-free rate plus a risk premium related to the company’s activity.
Applying the CAPM gives us:
where k is the weighted average cost of capital, rF the risk-free rate, rM the market rate of return and βA the beta of assets or unlevered beta; that is, the β of a debt-free company.
Just as the beta of a share measures the deviation between its returns and those of the equity market, so too does the beta of an asset measure the deviation between its future cash flows and those of the market. Yet these two betas are not independent. A firm that invests in projects with a high βA – in other words, projects that are risky – will have a high βE on its shares because its profitability will fluctuate widely.
On average, asset betas are below 1 as it is equity betas that are on average, by construction, equal to 1. Excluding the burden of net debt (which is on average positive for firms), asset betas are lower than equity betas.
Source: Data from BNP Paribas, Corporate Finance, team BVT, 2021
The βA can easily be computed, knowing that it is equal to the weighted average of the β of equity and the β of debt, with the weighting being a function of the relative importance of shareholders’ equity and net debt by value (V) in the company’s financial structure:
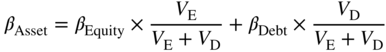
βA can also be expressed as follows:
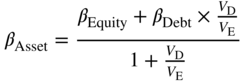
βDebt corresponds to the beta of the net debt and it should be computed exactly the same way as the beta of equity, which is by regressing the returns on listed debt against market returns. However, it is reasonable to assume that βDebt is equal to zero for weakly leveraged companies. Thus, the previous equation can be simplified as follows:
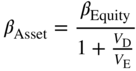
We believe that it is not reasonable to simplify the analysis by assuming that βDebt = 0 if the leverage of a company is not negligible. In fact, the higher the leverage, the less the financial debt depends on the level of interest rates and the more will be linked to the specific characteristics of the company (fixed costs/variable costs) and its industry (cyclicality). In these cases, debt then begins to behave more like equity in terms of beta characteristics.
Often, our readers will read that financial analysts prefer using the following formula:
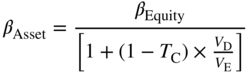
This formula differs from the one above, not only as βDebt is assumed to be nil but also because corporate income tax rate (TC) is introduced. The link between βAsset and βEquity is still subject to debate and depends on the assumptions made regarding the change in capital structure.
This way of computing βAsset is based on the work of F. Modigliani and M. Miller in 1963 (see Section 33.1), according to which the value of the firm is equal to its unlevered value plus the value of the tax shield of debt, computed as the product of the net debt multiplied by the corporate tax rate.
However, this assumes that the firm’s indebtedness is constant in perpetuity (which is rarely the case), that the tax rate is constant over time (which has been systematically untrue for decades), that financial interests are fully tax deductible (which is not the case when they become high) and that the cost of bankruptcy is negligible. Actually, for firms with some debts, cost of bankruptcy is of the same order of magnitude as the value of the tax saving due to the debt (Section 33.1).That is a lot for us to embrace!
2/ INDIRECT CALCULATION
Since we are looking for the return globally required by all the company’s providers of funds, it is possible to reconstruct the cost of capital based on the valuation of the various financial securities issued by the company.
Linked to the value of all its securities, the cost of capital represents the cost that the company would have to pay today to raise capital so as to replenish all its financial resources, regardless of the accounting costs of the resources its currently uses. It thus represents the introduction of the logic of the financial market within the company.
Consequently, it is possible to reconstitute the cost of capital of a company by seeking which rate of return is required for each type of financial security and by weighting each rate by its relative share in value in the financing of the company. The result is the weighted average cost of capital formula:
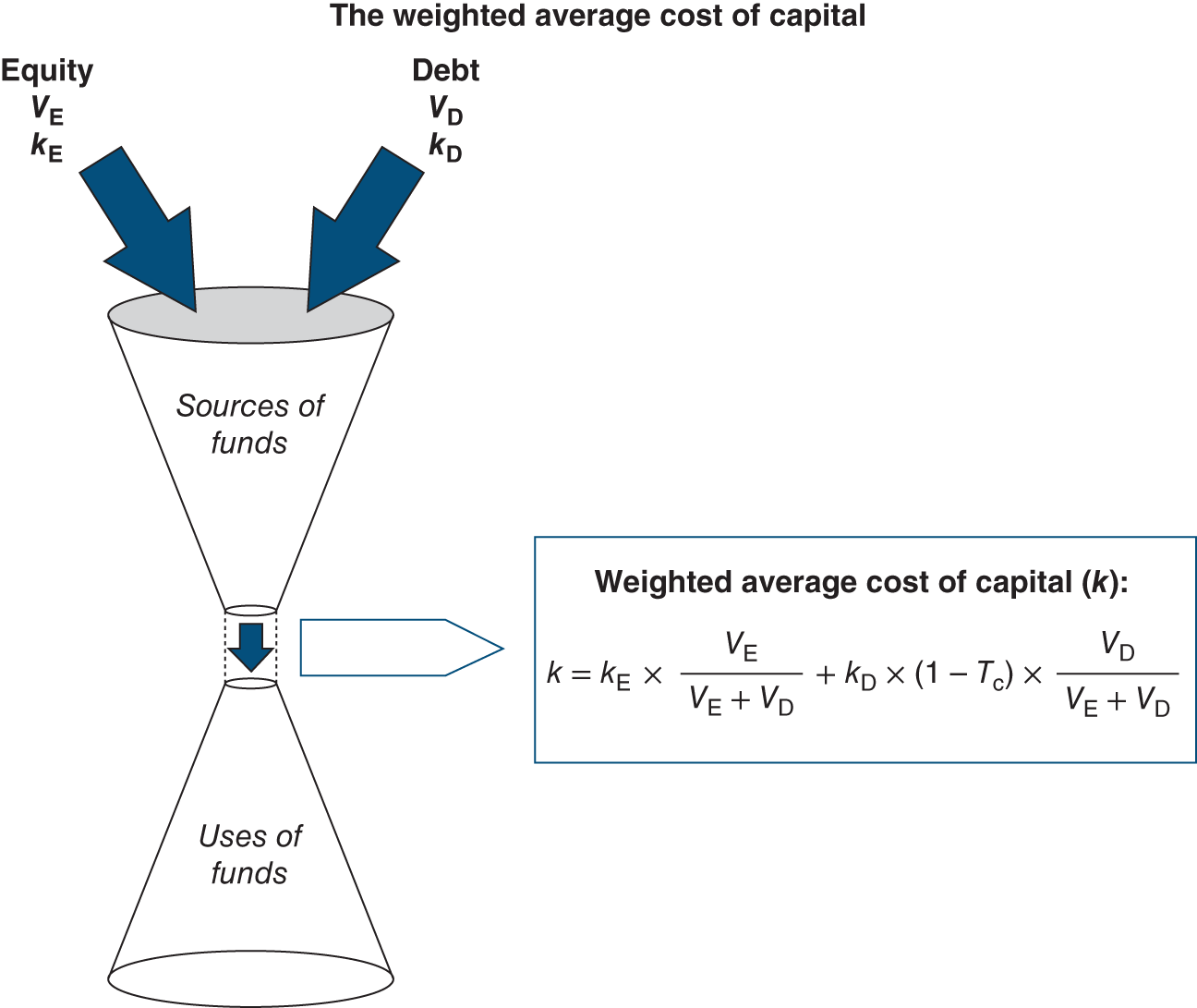
Thus, a company with equity financing of 100 at a rate of 8%, and debt financing of 50 at a pre-tax cost of 3%, has a cost of capital of 6.1% (with a 25% tax rate, Tc).
This is the most frequently used method to calculate the cost of capital. Nevertheless, beware of relying too much on spreadsheets to calculate the cost of capital, instead of getting your hands dirty by working on some examples yourself.
When performing simulations, it is all too tempting to change the company’s capital structure while forgetting that the cost of equity and the cost of debt are not constant: they are a function of the company’s structure. It is all too easy to fictitiously reduce the cost of capital on paper by increasing the relative share of net debt, because debt is always cheaper than equity!
In the preceding example, if the share of debt is increased to 80% without changing either the cost of debt or equity, then the cost of capital works out to be 3.4%. While the arithmetic may be correct, this is totally wrong financially.
Do not forget that higher debt translates into:
- a higher cost of equity, as βEquity increases with the debt level, as shown in the graph for food companies,
- a higher cost of debt, as the cost of debt increase with the debt level (Section 20.2).
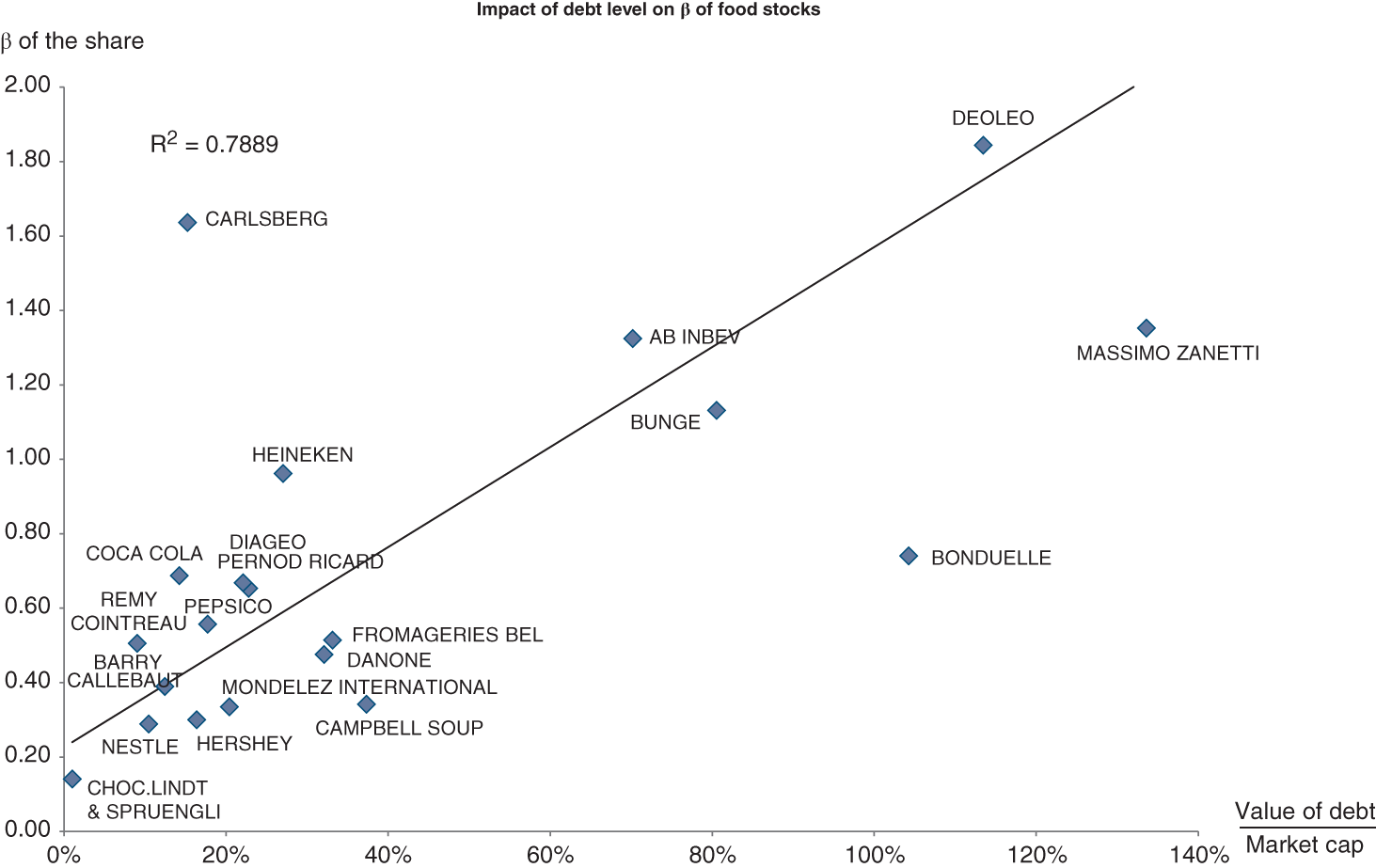
Source: Data from Barra, Factset, annual reports
3/ THE IMPLICIT CALCULATION BASED ON ENTERPRISE VALUE
The cost of capital can be estimated based on enterprise value and a projection of anticipated future free cash flows, since:
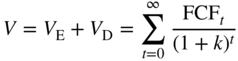
It is then necessary to solve the equation with k as the unknown factor. However, this calculation is rarely used because it is difficult to determine the market consensus for free cash flows over a long period of time.
4/ THE PITFALLS OF THE INDIRECT COST-OF-CAPITAL CALCULATION
(a) Expected rate of return or effective rate of return?
The cost of capital is a financial concept reflecting the expected rate of return required or expected by investors at a given point in time. It is not an accounting concept and should not be confused with the ex-post return on capital employed, which is the effective rate of return.
We won’t teach our readers that there is often a big difference between what we wish for and what we get.3
The cost of capital is neither an inverted P/E, nor the return on equity (ROE), nor the rate of return. Instead, it is the rate of return currently required by shareholders as measured by the CAPM:
The cost of debt is not the cost of debt contracted 10 years, one year or three months ago. Nor is it the company’s average cost of debt or the ratio of financial expenses to average debt for the year, as studied in Chapter 12, which covered the nominal cost of debt.
(b) Accounting or market values of equity and debt?
Shareholders base their required rate of return on the market value of equity; that is, the amount at which equity can be bought or sold, rather than using book values. The same reasoning applies to debtholders.
This is consistent with the idea of selecting the required rate of return rather than the book rate of return. Using the book value of equity and liabilities can be very misleading, because it may significantly differ from the market value of equity and debt.
For example, the Nestlé shareholder does not require a 27%4 return on book equity of CHF 46bn, but a 5% return on market capitalisation of CHF 310bn! Similarly, an investor buying Legrand USD-denominated bonds with a nominal yield of 8.5% at a price of 127% of the nominal amount does not require an 8.5% return. Instead, they are looking for 1.08%.5
Section 29.3 SOME PRACTICAL APPLICATIONS
1/ FOR THE INVESTMENT DECISION
When making an investment decision, and even if using the indirect method, it is not particularly difficult to calculate the cost of capital. If the company is publicly listed, the calculation is based on readily available market data. Average prices are often used to smooth out any erratic market swings. If the company is not listed, the calculation is based on the cost of capital of companies of comparable size and risk operating in the same sector of activity and geographic region. If the peer sample has been well chosen, the resulting cost of capital will be the same as that of the unlisted company.
The trick is elsewhere; one should not mix up the cost of capital of the firm and the cost of capital of the project. The two are the same only if the risk level of the project is the same as that of the firm.
If the company is engaging in a greenfield project (e.g. a new oil field for an oil company), it should add to its cost of capital a premium6 of c. 2%.
The level or risk of a project can also evolve in time. Usually, the average WACC over the duration of the project will be retained. But it may be more accurate to use a different WACC for each period depending on the maturity and therefore the risk of the investment.
If the company invests in a new sector or a new geography, it will not be able to use its current cost of capital to assess the project. The risk of the project will have to be taken into account to determine the cost of capital to be used. The cost of capital will therefore reflect the industry and geographic risk of the project.
The cost of the funds that will be used to finance the project should never be treated as the cost of capital.
If the financing is by debt, the rate of return to be demanded is certainly higher than the cost of the debt, since the equity, which is used to guarantee the lenders, and without which lenders would not lend, must be remunerated.
Alternatively, if the project is financed by new equity, the cost of capital to be used is likely to be lower than the cost of equity as the higher overall equity will reduce the risk borne by debt holders, and hence their remuneration.
Retaining the cost of the financing source directly instead of the cost of capital will lead to erroneous investment choices, as illustrated by the following example.
Let’s take a first investment with an IRR of 8% to be financed with equity that yields a cost of 10%. As the return of the investment does not cover its cost of financing, it is rejected.
A second investment with a similar risk has an IRR of 6%, this time to be financed with debt costing 4%. This investment will then be undertaken as its return is above the cost of financing.
As a result, this reasoning has led the company to undertake the investment yielding the lower return (6% vs. 8%) for the same level of risk. This clearly shows that the reasoning is flawed!
2/ CONCERNING ENERGY AND SOCIAL TRANSITION INVESTMENTS
Contrary to what we have just written in the previous paragraph, we believe that when a company is capable of raising funds via green, social or sustainable bonds or bank facilities whose cost depends on certain environmental or social criteria (see Section 20.4 and Section 21.2), the cost savings that investors may grant should be fully reflected in the cost of capital of the project. Helping the planet is well worth a departure from dogma!
This being said, even when savings do occur, they are relatively marginal in nature.
3/ FOR VALUATION
The indirect method is less adapted to valuations, because to determine the value of equity one needs the cost of capital (see Chapter 31), and to calculate the cost of capital one needs the value of equity! However, there are three ways to solve this dilemma:
- use the parameters associated with a target capital structure, that the firm should reach in a few years. While being careful to use the costs of equity and net debt that correspond to the target capital structure, and not the present costs. This is unfortunately a frequently committed error;
- state the equation of the value of equity (knowing that you need the value of equity to derive the discount rate) and find, by successive approximation, the discount rate that fits. Excel does iterative calculations that will solve this issue;
- use the direct method: the advantage of this alternative is that one avoids the frequent mistake of using costs of equity and debt that do not correspond to the capital structure in question.
4/ DIVERSIFIED COMPANIES
Thus Berkshire Hathaway’s cost of capital in itself makes little sense, it is only an a posteriori average of the cost of capital of an insurance group (Geico), a railway company (BNSF), a food company (Kraft Heinz), a portfolio of minority stakes in listed groups (Apple, Bank of America, etc.), etc.
As shown in Chapter 26, diversification does not reduce the cost of capital because it only considers systematic risk. As unsystematic risk can be eliminated by diversification; it does not affect the required rate of return and therefore has no impact on cost of capital.
5/ MULTINATIONAL COMPANIES
A similar logic applies to companies operating in different countries.
A British company investing in Russia, for example, should not use a discount rate based on British data just because its suppliers of funds are British.
After all, the project’s flows are affected by the Russian systematic risks (inflation, taxation, exchange rates, etc.) rather than the British systematic risk. It is therefore necessary to take a Russian risk-free rate, to select the βAsset to which a Russian risk premium is applied, to take into account the cost of Russian debt and in no case the UK parameters. This approach avoids the frequent error of discounting flows denominated in a given currency using a discount rate expressed in another currency.
A British, German or Chinese company wanting to invest in the exact same asset in Russia will bear the same cost of capital as it only depends on the risks of the project and not on the risks of the company investing.
After the Western-based company has invested in Russia, its cost of capital will probably be higher as it will be made up of two costs, a lower one for Western Europe and a higher one for Russia, reflecting the different levels of systemic risk (political and macroeconomic) in the two regions.
6/ EMERGING MARKETS
In developing countries, calculating the cost of capital of an investment raises some practical problems. The risk-free rate of local government bonds is often just wishful thinking, since these countries have little solvency. The local risk-free rate and betas of local peer groups are rarely measured, let alone significant, given the limited size of financial markets in these countries.
We suggest Bancel and Perrotin’s (1999) system for calculating the cost of capital in such cases:
The sovereign spread represents the difference between bond yields issued on international markets (in euros or dollars) by the country in question versus those offered by euro- or dollar-zone bonds. This yield represents the political risk in the emerging country. When the developing nation has not made any international issues, it is possible to use a bond issue by another state with the same credit rating as a benchmark.
When the sovereign spread reflects the fact that the state cannot be considered a risk-free borrower (like Venezuela), we advise using the spread of the best-rated borrower.
βA is the beta coefficient of the sector of activity calculated in developed financial markets. This parameter measures the sensitivity of an industry’s flows to the overall economic environment. It is shaped by the sector of activity, not the country.
Obviously, this rate must be applied to flows that have been converted from their local currencies into euros. If the flows are denominated in dollars, then remember to apply a USD rather than a euro benchmark.
For example, it is possible to calculate the cost of capital of a South African investment project based on the following assumptions: βA = 0.82, rF in the US = 3.0%, a South African government bond rate of 5.3% (bonds denominated in USD), a US risk premium of 6%.
If the project’s flows are denominated in South African rands, then the cost of capital is converted from dollars into rands as follows:
This assumes that the rand devaluates against the dollar regularly, in line with the inflation rate differential (purchase power parity).
7/ COMPANIES WITH NEGATIVE NET FINANCIAL DEBT
Consider a group that, for structural reasons, has a negative net debt of 2 with no banking or financial debt, and equity of 9.
Assume that the shareholders buying these shares understand that they are buying both operating assets with a given risk level and have a cash situation with virtually no risk. In other words, the risk on the share is lower than the risk on the company given the structurally positive net cash balance.
The cost of capital of this company can be estimated using the indirect method, applying a negative value for VD. So, in this example, if the cost of equity is 7% and net cash generates 2% after taxes, then the company’s cost of capital is 8.4%:
To offer the 7% return required by shareholders, the company must invest in projects yielding at least 8.4%. The 7% cost of equity is the weighted average of the required 8.4% return on capital employed and the 2% on net cash.
Practitioners often use a cost of capital equal to the cost of equity when the firm holds net cash. This is a mistake, unless you consider that shareholders do not take into account the security brought by the net cash.
8/ COMPANIES IN FINANCIAL DISTRESS
It is generally assumed that companies under financial distress have a very high cost of capital. This is not correct! Bankruptcy risk is a specific risk and not a systematic risk, and it should therefore not be taken into account by the cost of capital. If things were not so, the firm in financial distress could never undertake an investment as it would require a higher return than other firms in the sector. It could then never recover.
On the other hand, its cost of equity can be very high (βEquity between 3 and 10 can be observed), as the value of equity has become negligible compared to the value of debt. But as equity weighs very little in the capital structure, the influence of cost of equity on cost of capital is minimal.
Section 29.4 CAN CORPORATE MANAGERS INFLUENCE THE COST OF CAPITAL?
Chapters 32 and 33 demonstrate why there is little point in using debt and its tax advantages to lower the cost of capital. While net debt costs less than equity, it tends to increase the risk to shareholders, who retaliate by raising the required rate of return and consequently the cost of equity. Debt works to the advantage of the company, because the interest on the net debt can be deducted from its tax base (which it cannot do for dividends). The opposite tends to apply to investors.
In short, in a perfect world in which investors had diversified portfolios, one man’s gain would be another man’s loss.
Moreover, if debt really did reduce the cost of capital, one would have to wonder why highly efficient companies – such as L’Oréal, Heineken, Toyota, Google and SAP – are not levered, given that they have no reason to fear bankruptcy.
Since the cost of capital depends on the risk to the company, the only way it can be lowered is through risk-reducing measures, such as:
- Lowering the breakeven point by shifting from fixed to variable costs, i.e. subcontracting, outsourcing, etc. Unfortunately, the margins will probably decline accordingly.
- Improving the business’s visibility and smoothing its cyclical nature, i.e. winning medium-term supply contracts with important clients. Here too, however, margins may be affected since, in exchange, the clients will demand price concessions.
- Diversifying the business does not help as it does not reduce market risk, but rather specific risk, which is the only one to be remunerated.
- Shifting from a risky activity (e.g. a biotech start-up) in a high-risk country like Pakistan to a safer business in a more stable country (cheese production in Switzerland) will no doubt cut the cost of capital, but it will also lower profitability. In addition, it would have no impact on value, since it is simply a move on the security market line.
Similarly, increasing the risk for capital employed increases the cost of capital, but value will not be destroyed if profitability improves at the same time.
The cost of capital of Bouygues increased as it launched media and telecom activities (riskier than its traditional construction operations) and then decreased as these operations matured.
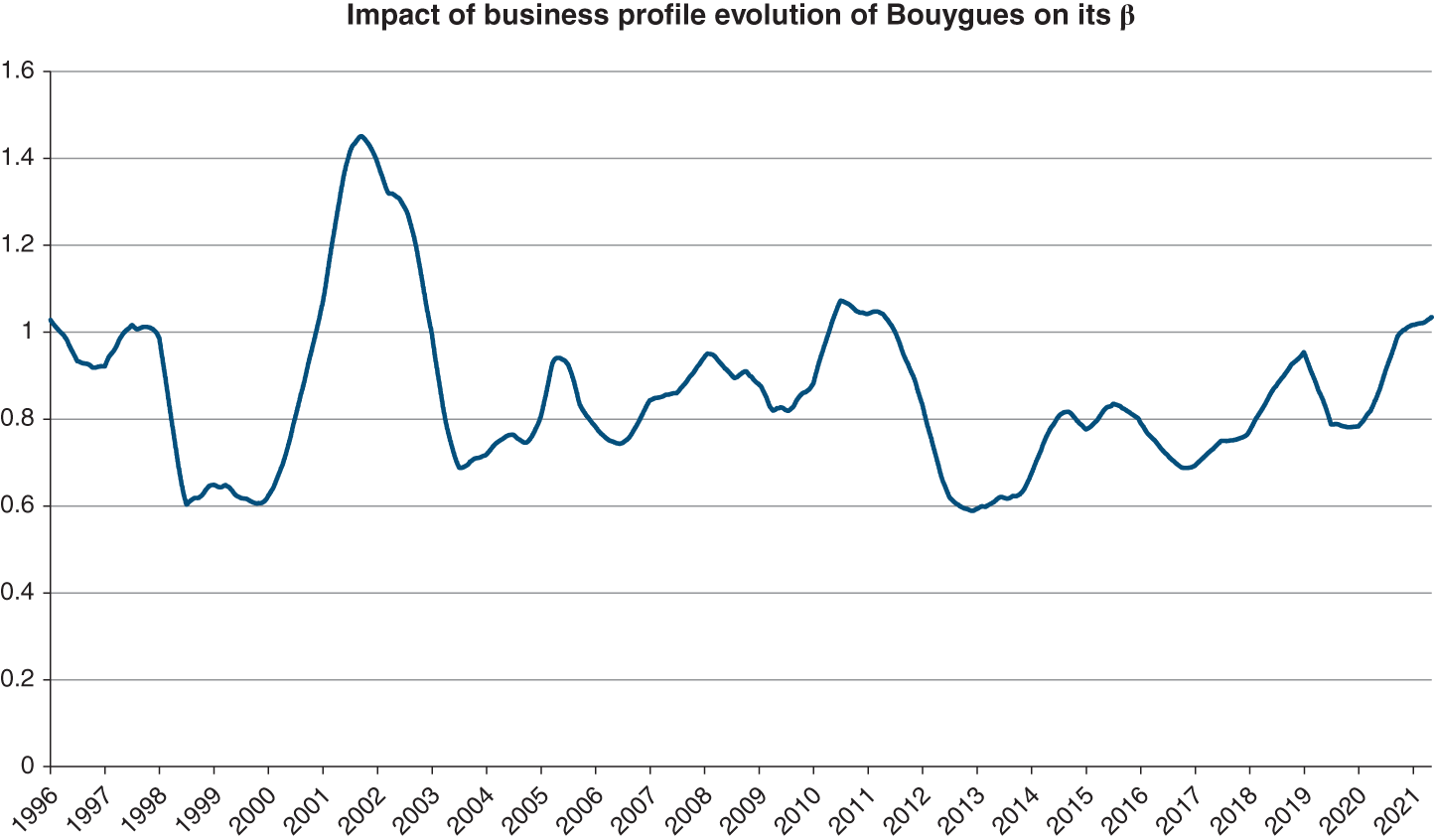
Source: Data from Factset, moving average over 6 months
SUMMARY
QUESTIONS
EXERCISES
ANSWERS
BIBLIOGRAPHY
NOTES
- 1 See Chapters 32 and 33.
- 2 This figure is lower than 1 since it is not the β of all shares on the market that average 1, but the β excluding the impact of net debt.
- 3 If necessary, we direct our readers to the Rolling Stones’ “You can’t always get what you want”.
- 4 Book return on 2020 equity.
- 5 Yield to maturity of the bond listed at 127% and maturing 2025.
- 6 Called greenfield premium.
- 7 See Easley and O’Hara (2004).
Chapter 30. RISK AND INVESTMENT ANALYSIS
When uncertainty creates value …
Valuing an investment by discounting future free cash flows at the weighted average cost of capital can provide some useful parameters for making investment decisions, but it does not adequately reflect the investors’ exposure to risk. On its own, this technique does not take into account the many factors of uncertainty arising from non-financial investments.
As a result, many risk analysis methods have been developed. However, these methods have limitations that only a radically different approach seeks to overcome. We will see that the application of option theory to investment choice theoretically offers this possibility. Although it brings decisive concepts to risk analysis in investment, it is far from being frequently applied, often for good reasons.
Section 30.1 ASSESSING RISK THROUGH THE BUSINESS PLAN
1/ BUILDING A BUSINESS PLAN
The reader must realise that the business plan is the first stage in assessing the risks related to an investment. The purpose of the business plan is to model the firm’s most probable future, and it helps to identify the parameters that could significantly impact on a project’s value. For example, in certain industries where sales prices are not very important, the model will be based on gross margins, which are more stable than turnover.
Establishing a business plan helps to determine the project’s dependence upon factors over which investors have some influence, such as costs and/or sales price. It also outlines those factors that are beyond entrepreneur’s control, such as raw material prices, exchange rates, etc. Obviously, the more the business plan depends upon exogenous factors, the riskier it becomes.
2/ SENSITIVITY ANALYSIS
One important risk analysis consists of determining how sensitive the investment is to different economic assumptions. This is done by holding all other assumptions fixed and then observing the impact of each economic assumption on the present value. It is a technique that highlights the consequences of changes in prices, volumes, rising costs or additional investments on the value of projects.
Firms generally build three scenarios (pessimistic, realistic and optimistic). In certain sectors highly dependent on raw materials or other exogenous factors (such as the price of electricity), investment scenarios are deduced from predetermined macroeconomic scenarios.
The sensitivity analysis requires a good understanding of the sector of activity and its specific constraints. The industrial analysis must be rounded off with a more financial analysis of the investment’s sensitivity to the model’s technical parameters, such as the discount rate or terminal value (perpetuity growth rate, see Chapter 31).
Practitioners usually build a sensitivity matrix, which offers an overview of the sensitivity of the investment’s NPV to the various assumptions.
Other companies prefer to focus on only one scenario, which is analysed in depth in order to keep managers of the project committed.
3/ ASSESSMENT OF THE MAXIMUM RISK
Investors, in particular if they are not familiar with the sector (which is usually the case for financial investors), may be tempted to build a very pessimistic scenario (worst-case scenario or crash test). Nevertheless, this scenario needs to remain realistic and thus cannot be a cumulative sensitivity analysis.
This exercise does not aim to determine a value but rather to assess the risk of failure (and potentially bankruptcy) of the project or to assess the additional investments that would then be needed. This scenario can also be useful to fix the maximum level of debt that the project can take.
Section 30.2 ASSESSING RISK THROUGH A MATHEMATICAL APPROACH
1/ MONTE CARLO SIMULATION
An even more elaborate variation of scenario analysis is the Monte Carlo simulation, which is based on more sophisticated mathematical tools and software. It consists of isolating a number of the project’s key variables or value drivers, such as turnover or margins, and allocating a probability distribution to each. The analyst enters all the assumptions about distributions of possible outcomes into a spreadsheet. The model then randomly samples from a table of predetermined probability distributions in order to identify the probability of each result.
Assigning probabilities to the investment’s key variables is done in two stages. First, influential factors are identified for each key variable. For example, with turnover, the analyst would also want to evaluate sales prices, market size, market share, etc. It is then important to look at available information (long-run trends, statistical analysis, etc.) to determine the uncertainty profile of each key variable using the values given by the influential factors.
Generally, there are several types of key variables, such as simple variables (e.g. fixed costs), compound variables (e.g. turnover = market × market share) or variables resulting from more complex, econometric relationships.
The investment’s net present value is shown as an uncertainty profile resulting from the probability distribution of the key variables, the random sampling of groups of variables and the calculation of net present value in this scenario.
Repeating the process many times gives us a clear representation of the NPV risk profile.
Once the uncertainty profile has been created, the question is whether to accept or reject the project. The results of the Monte Carlo method are not as clear cut as present value, and a lot depends upon the risk/reward trade-off that the investor is willing to accept. One important limitation of the method is the analysis of interdependence of the key variables; for example, how developments in costs are related to those in turnover.
2/ THE CERTAINTY EQUIVALENT
The certainty equivalent of a future cash flow is the certain amount that the investor would be ready to accept in exchange for an expected future risky cash flow. For example, if the investor is expecting a project to provide a 1,000 cash flow in one year, given the risk they may consider trading this cash flow for the certainty of getting 600 in one year.
The certainty equivalent method leads to discounting using the risk-free rate and the certainty equivalent cash flows. The net present value of an investment can then be written as:

where ei is the certainty equivalent factor of cash flow CFi and rF the risk-free rate.
To the best of our knowledge, this method remains rarely used in practice.
Section 30.3 THE CONTRIBUTION OF REAL OPTIONS
1/ THE LIMITS OF CONVENTIONAL ANALYSIS
Do not be confused by the variety of risk analysis techniques presented in the preceding section. In fact, all of these different techniques are based on the same principle. In the final analysis, simulations, the Monte Carlo or the certainty equivalent methods are just complex variations on the NPV criteria presented in Chapter 16.
Like NPV, conventional investment risk analyses are based on two fundamental assumptions:
- the choice of the anticipated future flow scenario; and
- the irreversible nature of the investment decision.
The second assumption brings up the limits of this type of analysis. Assuming that an investment is irreversible disregards the fact that corporate managers, once they get new information, generally have a number of options. They can abandon the investment halfway through if the project does not work out, they can postpone part of it or extend it if it has good development prospects, or they can use new technologies. The teams managing or implementing the projects constantly receive new information and can adapt to changing circumstances. In other words, the conventional approach to investment decisions ignores a key feature of many investment projects, namely flexibility.
It might be argued that the uncertainty of future flows has already been factored in via the expected value criterion and the discount rate, and therefore this should be enough to assess any opportunities to transform a project. However, it can be demonstrated that this is not necessarily so.
2/ REAL OPTIONS
Entrepreneurs are not just passively exposed to risks. In many cases, they are able to react to ongoing events. They can increase, reduce or postpone their investment, and they exercise this right according to ongoing developments in prospective returns.
In fact, the entrepreneur is in the same situation as the financial manager, who can increase or decrease his position in a security given predetermined conditions.
Entrepreneurs who have some leeway in managing an investment project are in the same position as financial managers holding an option.1
The flexibility of an investment thus has a value that is not reflected in conventional analysis. This value is simply that of the attached option. Obviously, this option does not take the form of the financial security with which you have already become familiar. It has no legal existence. Instead, it relates to industrial assets and is called a real option.
The potential flexibility of an investment, and therefore of the attached real options, is not always easy to identify. Industrial investors frequently do not realise or do not want to admit (especially when using a traditional investment criterion) that they do have some margin for manoeuvre. This is why it is often called a hidden option.
3/ REAL OPTIONS CATEGORIES
Given the potential value of hidden options, it is tempting to consider all investment uncertainties as a potential source of value. But the specific features of option contracts must not be overlooked. The following three factors are necessary to ensure that an investment project actually offers real options:
- The project must have a degree of uncertainty. The higher the underlying volatility, the greater the value of an option. If the standard deviation of the flows on a project is low, the value of the options will be negligible.
- Investors must be able to get more information during the course of the project, and this information must be sufficiently precise to be useful.
- Once the new information has been obtained, it must be possible to change the project significantly and irrevocably. If the additional information cannot be used to modify the project, the manager does not really have an option but is simply taking a chance. In addition, the initial investment decision must also have a certain degree of irreversibility. If it can be changed at no cost, then the option has no value. And lastly, since the value of a real option stems from the investor’s ability to take action, any increase in investment flexibility generates value, since it can give rise to new options or increase the value of existing options.
Real options apply primarily to decisions to invest or divest, but they can appear at any stage of a company’s development. As a result, the review in this text of options theory is a broad outline, and the list of the various categories of real options is far from exhaustive.
The option to launch a new project corresponds to a call option on a new business. Its strike price is the start-up investment, a component that is very important in the valuation for many companies. In these cases, they are not valued on their own merits, but according to their ability to generate new investment opportunities, even though the nature and returns are still uncertain.
By acquiring a controlling stake in Symbio in 2019, Michelin took an option on the future development of hydrogen as a means of powering vehicles, through the hydrogen-powered fuel cells Symbio develops.
Similarly, R&D departments can be considered to be generators of real options embedded within the company. Any innovation represents the option to launch a new project or product. This is particularly true in the pharmaceutical industry. If the project is not profitable, this does not mean that the discovery has no value. It simply means that the discovery is out of the money. Yet this situation could change with further developments.
The option to develop or extend the business is comparable to the launch of a new project. However, during the initial investment phase decisions have to be made, such as whether to build a large factory to meet potentially strong demand or just a small plant to test the waters first.
A real options solution would be to build a small factory with an option to extend it if necessary. Flexibility is just as important in current operations as it is when deciding on the overall strategy of a project. Investments should be judged by their ability to offer recurring options throughout their lifecycle. Certain power stations, for example, can easily be adapted to run on gas or oil. This flexibility enhances their value, because they can easily be switched to a cheaper source of energy if prices fluctuate. Similarly, some auto plants need only a few adjustments in order to start producing different models.
The option to reduce or contract business is the opposite of the previous example. If the market proves smaller than expected, the investor can decide to cut back on production, thus reducing the corresponding variable costs. Indeed, the investor can also decide not to carry out part of the initial project, such as building a second plant. The implied sales price of the unrealised portion of the project consists of the savings on additional investments. This option can be described as a put option on a fraction of the project, even if the investment never actually materialises.
The option to postpone a project. The initial investment to get the authorisations to build a wind farm is minimal in comparison with construction costs. It can thus be quite useful to defer the start of the project, for example until the business environment becomes more propitious (electricity prices, operating costs, etc.). To a certain extent, this is similar to holding a well-known but not fully exploited brand.
Nonetheless, the option to defer the project’s start is valid only if the investor is able to secure ownership of the project from the outset. If not, competitors may take on the project. In other words, the advantage of deferring the investment could be cancelled out by the risk of new market entrants.
Looking beyond the investment decision itself, option models can be used to determine the optimal date for starting up a project. In this case, the waiting period is similar to holding an American option on the project. The option’s value corresponds to the price of ensuring future ownership of the project (land, patents, licence, etc.).
The option to defer progress on the project is a continuation of the previous example. Some projects consist of a series of investments rather than just one initial investment. Should investors receive information casting doubt on a project that has already been launched, they may decide to put subsequent investments on hold, thus effectively halting further development. In fact, investors hold an option on the project’s further development at every call for more financing.
The option to abandon means that the industrial manager can decide to abandon the project at any time. Thus, hanging on to it today means keeping open the option to abandon at a later date. However, the reverse is not possible. This asymmetry is reflected in options theory, which assumes that a manager can sell the project at any time (but might not be able to buy it back once it is sold).
Such situations are analogous to the options theory of equity valuations that we will examine in Chapter 34. If the project is set up as a levered company, then the option to abandon corresponds to shareholders’ right to default. The value of this option is equal to that of equity, and it is exercised when the amount of outstanding debt is greater than the value of the project.
In the example below, the project includes an option to defer its launch (wait and see), an option to expand if it proves successful and an option to abandon it completely.

4/ EVALUATING REAL OPTIONS
Option theory sheds light on the valuation of real options by stating that uncertainty combined with flexibility adds value to an investment opportunity. How appealing! It tells us that the higher the underlying volatility, and thus the risk, the greater the value of an option. This appears counterintuitive compared with the net present value approach, but remember that this value is very unstable. The time value of an option decreases as it reaches its exercise date, since the uncertainty declines with the accumulation of information on the environment.
Consider the case of a software publisher that is offered the opportunity to buy a licence to market (after development) connected homes software for £5m. If the publisher does not accept the deal right away, the licence will be offered to a rival. The software can be developed on the spot at a cost of £50m.
If the software is developed immediately, the company should be able to generate £2m in cash flows over the next year. The situation the following year, however, is far more uncertain, since one of the main equipment suppliers for connected homes is due to choose a new technological standard. If the standard chosen corresponds to that of the licence offered to our company, it can hope to generate a cash flow of £9m per year. If another standard is chosen, the cash flows will plunge to £1m per year. The management of our company estimates there is a 50% chance that the “right” standard will be chosen. As of the second year, the flows are expected to be constant to infinity.
The present value of the immediate launch of the product can easily be estimated with a discount rate of 10%. The anticipated flows are 0.5 × 9 + 0.5 × 1 = £5m from the second year on to infinity. Assuming that the first year’s flows are disbursed (or received) immediately, that second-year flows are disbursed (or received) at the very beginning of the second year and so on, the present value is 5 / 0.1 + 2 = £52m for a total cost of 50 + 5 = £55m. According to the NPV criterion, the project destroys £3m in value and the company should reject the licensing offer.
This would be a serious mistake!
If it buys the licence, the company can decide to develop the software whenever it wants to and can easily wait a year before investing in production. While this means giving up revenues of £2m in the first year, the company will have the advantage of knowing which standard the equipment supplier will have chosen. It can thus decide to develop only if the standard is suited to its product. If it is not, the company abandons the project and saves on development costs. The licence offered to the company thus includes a real option: the company is entitled to earn the cash flows from the project in exchange for investing in development.
The NPV approach assumes that the project will be launched immediately. That corresponds to the immediate exercise of the call option on the underlying instrument. This exercise destroys the time value. To assess the real value of the licence, we have to work out the value of the corresponding real option, i.e. the option of postponing development of the software.
The value of an option can be determined by the binomial method, which we described in greater detail in Chapter 23.
Imagine that the company has bought the licence and put off developing the software for a year. It now knows what standard the carrier has chosen. If the standard suits its purposes, it can immediately start up development at an NPV of 9 × (1 + 1 / 0.1) − 50 = £49m at that date. If the wrong standard is chosen, the NPV of developing the software falls to 1 × (1 + 1 / 0.1) − 50 = −£39m, and the company drops the project (this investment is irreversible and has no hidden options). The value of the real option attached to the licence is thus £49m for a favourable outcome and 0 for an unfavourable outcome. Using a risk-free discount rate of 5%, the calculation for the initial value of the option is £23.3m, since:
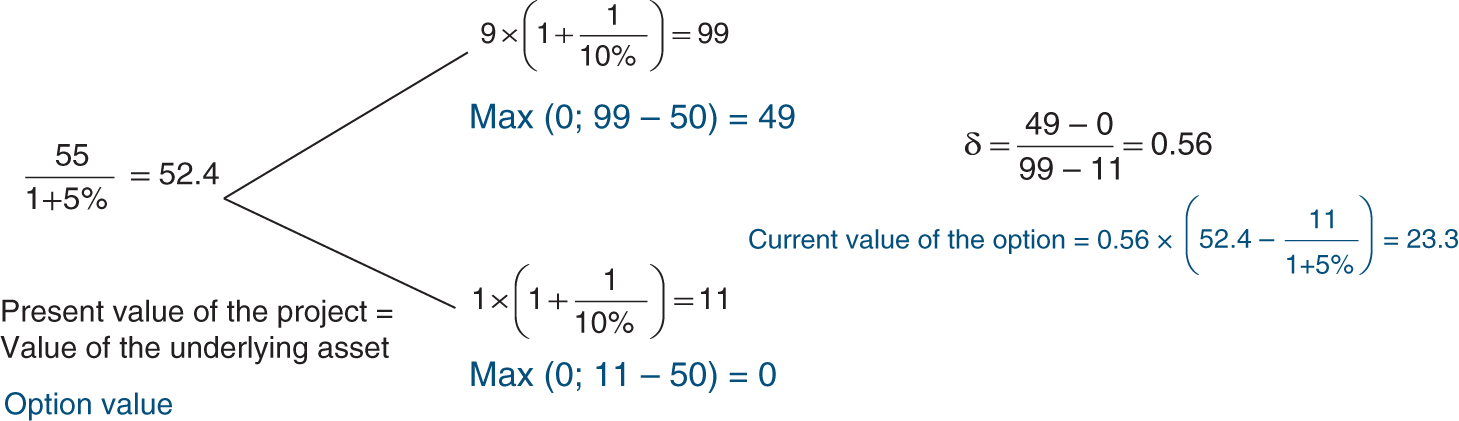
Here is another look at the licensing offer. The licence costs £5m and the value of the real option is £23.3m, assuming development is postponed for one year. With this proviso, the company has been offered the equivalent of an immediate gain of 23.3 − 5 = £18.3m.
In this example, the difference between the two approaches is considerable. Legend has it that when an oil concession was once being auctioned off, one of the bidding companies offered a price that was less than a tenth of that of its competitor, quite simply because he had “forgotten” to factor in the real options!
This example assumed just one binomial alternative but, when attempting to quantify the value of real options in an investment, one is faced by a myriad of alternatives. More generally, the binomial model uses the replicating portfolio approach, which requires the use of quite sophisticated mathematical tools. Estimating volatility is always a problematic issue with respect to the concrete application of this methodology. In addition, the method requires defining a convenience yield that represents the interest in holding an asset at a certain point in time given its expected return.
In practice, the information derived from the quantification of real options is frequently not very significant when compared with a highly positive NPV in the initial scenario. However, when NPV is negative at the outset, one always has to consider the flexibility of the project by resorting to real options.
5/ THE EXPANDED NET PRESENT VALUE
Since options allow us to analyse the various risks and opportunities arising from an investment, the project can be assessed as a whole. This is done by taking into account its two components – anticipated flows and real options. Some authors call this the expanded net present value (ENPV), which is the opposite of the “passive” NPV of a project with no options. ENPV is equal to the NPV grossed up with the value or real options of the investment.
When a project is very complex with several real options, the various options cannot be valued separately since they are often conditional and interdependent. If the option to abandon the project is exercised, then the option to reduce business obviously no longer exists and its value is nil. As a result, there is no additional value on options that are interdependent.
6/ CONCLUSION
The predominant appeal of real options theory is its factoring of the value of flexibility that the traditional approaches ignore. The traditional net present value approach assumes that there is only one possible outcome. It does not take into account possible adaptive actions that could be taken by corporate managers. Real options fill this gap.
But do not get carried away; applying this method can be quite difficult because:
- not everyone knows how to use the mathematical models. This can create problems in communicating findings; and
- estimating some of the required parameters, such as volatility, opportunity costs, etc., can be complicated.
We trust that the reader will not mind being told that the use of these tools by practitioners is inversely correlated to the place devoted to them in this chapter: virtually systematic for scenarios, less often for the Monte Carlo method and very rarely for real options.
Section 30.4 WHAT IF THE RISK WAS YOU?
Investment decisions in companies are rarely made by machines, but by human beings, who are naturally subject to cognitive biases, leading to systematic errors in reasoning without us really being aware of them. Their existence was highlighted by the research of psychologists and economists D. Kahneman and A. Tversky, who laid the foundations for the development of a new way of thinking called behavioural finance, which R. Thaler has strongly developed.
1/ COGNITIVE BIASES IN THE INVESTMENT DECISION
There are many such biases, both in the analysis phase of a project and in the decision phase. We will therefore only mention the main ones, which, as you will see, do not all point in the same direction.
Confirmation bias consists of seeing in the environment only the favourable elements that reinforce your conviction, those that you want to see and hear, you even actively seek them out; and neglecting, or even ignoring, those that contradict your thesis.
The risk aversion of managers tends to increase the further you get from the senior management of the company. Indeed, the success of a project will affect the career of an employee in charge of it much more than that of an executive directly and indirectly responsible for 10 projects, not to mention that of the ultimate leader whose personal future will depend on the success or failure of hundreds of investment projects. Here we find the notion of diversification. As a result, core managers will kill many projects, often with positive net present value, for fear that their possible failure will affect them strongly, while a success will be considered normal. We suggest that you answer question 12 in this chapter for an illustration, where you will see that most managers are more sensitive to a given loss than to a given success of the same amount.
The overconfidence bias or optimism bias that makes you ignore the response that your competitors are bound to have to the new product you are launching, which makes you confuse “managerial voluntarism with optimism about factors that are beyond the company’s control” (Sibony, 2020). As an investor, we do not remember seeing a pessimistic business plan! Question 13 of this chapter is for you.
The group bias that often makes those of a different opinion hesitate within a group and more often than not leads them to keep their objections quiet, when they could highlight neglected aspects that could prove crucial. This is regularly confirmed in the classroom, where the second student asked the same question will most often respond in the same way as the first student, let alone the third or fourth.
The interest bias that makes you defend your division’s investment project, because it is your division’s, making you forget to take into account the group’s interest, especially when it differs; or the investment banker who will not point out the negative elements of a merger that could dissuade his client from concluding it when his remuneration depends on it. To quote D. Ariely (2013): “We cheat to the extent that we can maintain the image of a reasonably honest person in our own eyes”.
2/ HOW TO LIMIT COGNITIVE BIASES IN THE INVESTMENT DECISION
In a study of 1,048 investment decisions, D. Lovallo and O. Sibony (2010) showed that the way in which the investment decision was made accounted for 53% of the variance in the profitability of the investment, compared with only 8% for the analyses of the investment. In other words, the way in which the investment decision is made explains 7 times more of its final profitability than its analysis itself.
Armed with this observation, and knowing that it is futile for an individual to try to identify and eliminate his cognitive biases, since by construction they are largely unconscious, O. Sibony (2020) makes three recommendations to improve investment decision-making and reduce the risk of failure:
- Organise dialogue to encourage the confrontation of points of view. The final decision-maker could thus ensure the diversity of skills and profiles of the members of the investment committee; take time for debate by blocking agendas for half a day or the day; clearly distinguish between projects submitted for discussion at this session and those submitted for decision, in order to avoid hasty conclusions and lack of maturation; give preference to the summary note rather than the Powerpoint presentation, which puts the debate to sleep and freezes it; Require project leaders to present several sets of projects; failing that, present an alternative story of why this project might be rejected or prepare a pre-mortem that describes a few years later why this project failed, in order to highlight the uncertainty inherent in decision-making; replace the management committee for decision-making with an ad-hoc committee of company executives from different functions who will escape the political games played within the exco. Thus informed, the final decision-maker will be able to make better decisions.
- Decentring to see the facts from another angle, and limit bias. The final decision-maker will thus maintain in the organisation, by promoting them, unconventional personalities who do not hesitate to systematically say what they think, maintain informal networks, call on experts, consultants, or even solicit another team in the company to deconstruct the investment project or solicit the opinion of employees by relying on the wisdom of crowds. And to avoid losing sight of the facts through the multiplication of contradictory points of view, the final decision-maker standardises the presentation formats, defines the decision criteria in advance, carries out stress tests on the hypotheses, and above all cultivates the humility that is the first quality of a good financier.
- Foster agility in decision-making. The final decision-maker will carry out real experiments, develop progressive commitments before making the final decision, reclaim the right to fail by first showing his own to evacuate the fear of failure in his collaborators. And the best advice is to make the final decision the morning after the final investment committee. It’s never clearer then!
SUMMARY
QUESTIONS
EXERCISE
ANSWERS
BIBLIOGRAPHY
NOTE
- 1 If you are not familiar with options, we advise you to read Chapter 23 before reading the rest of this chapter.
Chapter 31. VALUATION TECHNIQUES
That endless quest for fair value
Although you might not realise it, you already have the knowledge of all the tools that you will need to value a company. You discovered what discounting was about in Chapter 16 and learnt all about the right discount rate to use in Chapters 19 and 29. Finally, the comparable method was explained in Chapter 22. This chapter contains an in-depth look at the different valuation techniques and presents the problems (and solutions!) you will probably encounter when using them. Nevertheless, we want to stress that valuation is not a simple use of mathematical formulae, it requires the valuator to have good accounting and tax skills. You will also need to fully understand the business model of the firm to be valued in order to assess the reliability of the business plan supporting the valuation. Reading this chapter will only be a first step towards becoming a good valuator and, in addition, a great deal of practice and application will be needed.
Section 31.1 OVERVIEW OF THE DIFFERENT METHODS
Generally, we want to value a company in order to determine the value of its shares or of its equity capital.
Broadly speaking, there are two methods used to value equity: the direct method and the indirect method. In the direct method, obviously, we value equity directly. In the indirect method, we first value the firm as a whole (what we call “enterprise” or “firm” value), then subtract the value of net debt to get the equity value.
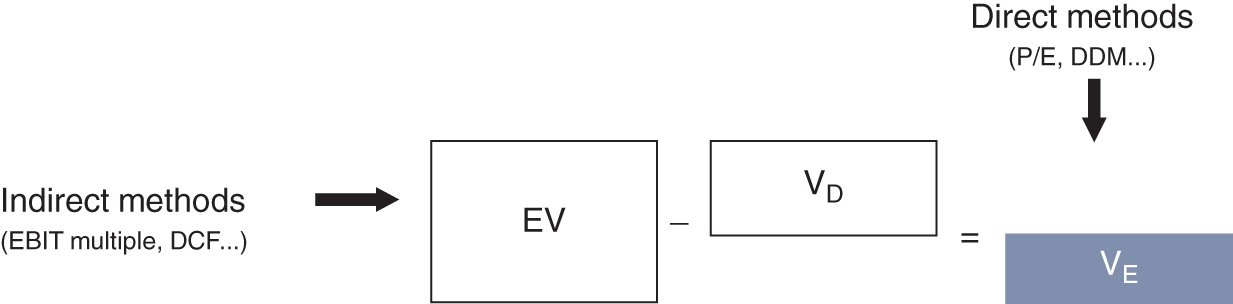
In addition, there are two approaches used in both the direct and indirect methods:
- The fundamental approach based on valuing either:
- a stream of dividends, which is the dividend discount model (DDM); or
- a stream of free cash flows, which is the discounted cash flow (DCF) method.
This approach attempts to determine the company’s intrinsic value, in accordance with financial theory, by discounting cash flows to their present value using the required rate of return.
- The pragmatic approach of valuing the company by analogy with other assets or companies of the same type for which a value reference is available. This is the peer comparison method (often called the comparables method). Assuming markets are efficient, we should be able to infer the value of a company from the value of others.
| Indirect approach | Direct approach | |
|---|---|---|
| Intrinsic value method (discounted present value of financial flows) | Present value of free cash flows discounted at the weighted average cost of capital (k) – value of net debt | Present value of dividends at the cost of equity: kE |
| Peer comparison method (multiples of comparable companies) | EBITDA/EBIT multiple × EBITDA/EBIT – value of net debt | P/E × net income |
The sum-of-the-parts method consists of valuing the company as the sum of its assets less its net debt. However, this is more a combination of the techniques used in the direct and indirect methods rather than a method in its own right.
Lastly, we mention options theory, whose applications we have seen in Chapter 30 and will see in Chapter 34. In practice, very few will value equity capital by analogy to a call option on the assets of the company. The concept of real options, however, had its practical heyday at the height of the stock market folly of 1999 and 2000, to explain the market values of “new economy” stocks. Needless to say, this method has since fallen out of favour (but may come back to justify the valuation of some tech groups today).
If you remember the efficient market hypothesis, you are probably asking yourself why market value and discounted present value would ever differ. In this chapter we will take a look at the origin of the difference, and try to understand the reason for it and how long we think it will last. Ultimately, market values and discounted present values should converge.
Section 31.2 VALUATION BY DISCOUNTED CASH FLOW
The discounted cash flow method (DCF) consists of applying the investment decision techniques (see Chapter 16) to the firm value calculation. We will focus on the present value of the cash flows from the investment. This is the fundamental valuation method (or intrinsic method). Its aim is to value the company as a whole (i.e. to determine the value of the capital employed, what we call enterprise value). After deducting the value of net debt, the remainder is the value of the company’s shareholders’ equity.
As we have seen, the cash flows to be valued are the after-tax amounts produced by the firm. They should be discounted out to perpetuity at the company’s weighted average cost of capital (see Chapter 29):
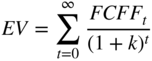
In practice, we project specific cash flows over a certain number of years. This period is called the explicit forecast period. The length of this period varies depending on the sector. It can be as short as two to three years for a high-tech company, five to seven years for a consumer goods company and as long as 20 to 30 years for a utility. For the years beyond the explicit forecast period, we establish a terminal value.
1/ SCHEDULE OF FREE CASH FLOWS
Free cash flows measure the cash-producing capacity of the company. Free cash flows are estimated as follows:
You buy a company for its future, not its past, no matter how successful it has been. Consequently, future cash flows are based on projections contained in the business plan of the company. As they will vary depending on growth assumptions, the most cautious approach is to set up several scenarios. But for starters, are you the buyer or the seller? The answer will influence your valuation. The objective of negotiation is to reconcile the buyer’s and seller’s points of view. We have found in our experience that discounted cash flow analysis is a very useful discussion tool: the seller gets accustomed to the idea of selling their company and the buyer gets a better understanding of the company for sale.
It is all right for a business plan to be optimistic – our bet is that you have never seen a pessimistic one – the important thing is how it stands up to scrutiny. It should be assumed that competition will ultimately eat into margins and that increases in profitability will not be sustained indefinitely without additional investment or additional hiring. Quantifying these crucial future developments means entering the inner sanctum of the company’s strategy.
(a) Business plan horizon
The length of the explicit forecast period will depend on the company’s “visibility” – i.e. the period of time over which is it reasonable to establish projections. This period is necessarily limited. In 10 years’ time, for example, probably only a small portion of the company’s profits will be derived from the production facilities it currently owns or from its current product portfolio. The company will have become a heterogeneous mix of the assets it has today and those it will have acquired over the next 10 years.
The forecast period should therefore correspond to the time during which the company will live off its current configuration. If it is too short, the terminal value will be too large and the valuation problem will only be shifted in time. Unfortunately, this happens all too often. If it is too long (more than 10 years), the explicit forecast is reduced to an uninteresting theoretical extrapolation.
Let’s look at ArcelorMittal’s financial projections and its stock trend chart. Below are a few projections that we made for teaching purposes, building on analysts’ consensus.
It should be noted that the ROCE at the end of the period (5.6%) is much lower than the cost of capital (10%) but on average over the period 2021–2025 (9.2%) it is quite close.
Projected after-tax free cash flows are as follows:
| ($m) | 2020 | 2021e | 2022e | 2023e | 2024e | 2025e |
|---|---|---|---|---|---|---|
| EBIT | 1,341 | 12,472 | 6,578 | 4,323 | 4,123 | 3,961 |
| − Corporate income tax at 25% | 335 | 3,118 | 1,645 | 1,081 | 1,031 | 990 |
| + Depreciation and amortisation | 2,960 | 2,536 | 2,536 | 2,789 | 2,800 | 2,912 |
| − Capital expenditure | 2,439 | 2,900 | 3,000 | 3,000 | 3,075 | 3,152 |
| − Changes in working capital | –1,841 | 1,395 | 322 | 67 | 75 | 91 |
| = Free cash flow | 3,368 | 7,595 | 4,148 | 2,964 | 2,742 | 2,640 |
Using a weighted average cost of capital of 10%, the end-2020 present value of the free cash flows generated during the explicit forecast period (2021–2025) is $16,071m.
Some practitioners discount cash flows over half years; the formula then becomes:
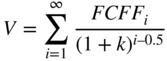
This assumes that cash flows are cashed in “on average” at half-year and that the valuation is performed at the beginning or end of the year.
(b) Terminal value
It is very difficult to estimate a terminal value because it represents the value at the date when existing business development projections will no longer have any meaning. Often analysts assume that the company enters a phase of maturity after the end of the explicit forecast period. In this case, the terminal value can be based either on the capital employed or on the free cash flow in the last year of the explicit forecast period.
The most commonly used terminal value formula is the Gordon–Shapiro formula. It consists of a normalised cash flow, or annuity, that grows at a rate (g) out to perpetuity:
Value of the company at the end of the explicit forecast period
However, the key challenge is in choosing the normalised free cash flow value and the perpetual growth rate. The normalised free cash flow must be consistent with the assumptions of the business plan. It depends on long-term growth, the company’s investment strategy and the growth in the company’s working capital. Lastly, normalised free cash flows may be different from the free cash flow in the last year of the explicit forecast period, because normalised cash flow is what the company will generate after the end of the explicit forecast period and will continue to generate to perpetuity.
Concerning the growth rate to perpetuity, do not get carried away:
- Apart from the normalised cash flow’s growth rate to perpetuity, you must take a cold, hard look at your projected long-term growth in return on capital employed. How long can the economic profit it represents be sustained? How long will market growth last?
- Most importantly, the company’s rate of growth to perpetuity cannot be significantly greater than the long-term growth rate of the economy as a whole. For example, if the anticipated long-term inflation rate is 2% and real GDP growth is expected to be 2%, then if you choose a growth rate g that is greater than 4%, you are implying that the company will not only outperform all of its rivals but also eventually take control of the economy of the entire country or indeed of the entire world (trees do not grow to the sky)!2
In the case of ArcelorMittal, the normalised cash flow must be calculated for the year 2026, because we are looking for the present value at the end of 2025 of the cash flows expected in 2026 and every subsequent year to perpetuity. Given the necessity to invest if growth is to be maintained, you could use the following assumptions to determine the normalised cash flow:
Using a rate of growth to perpetuity of 2%, we calculate a terminal value of \$35,095m. Discounted over five years, this gives us \$21,791m at the end of 2020. The enterprise value of ArcelorMittal is therefore \$16,071m + \$21,791m, or \$37,863m. Note that the terminal value of \$35,095m at end-2025 corresponds to a multiple of 8.9 times 2025 EBIT. This means that choosing a multiple of 8.9 is theoretically equivalent to applying a growth rate to perpetuity of 2% to the normalised cash flow and discounting it at the required rate of return of 10%.
Given a net debt of \$14,000m, the equity value of ArcelorMittal as at end-2020 works out, with this method, at \$23,863m.
Sometimes the terminal value is estimated based on a multiple of a measure of operating performance. This measure can be, among other things, turnover, EBITDA or EBIT. Generally, this “horizon multiple” is lower than an equivalent, currently observable, multiple. This is because it assumes that, all other things being equal, prospects for growth decrease with time, commanding a lower multiple. Nevertheless, since using this method to assess the terminal value implies mixing intrinsic values with comparative values, we strongly advise against it.
Computing the terminal value with a multiple prevents you from pondering over the level of ROCE that the company can maintain in the future.
Remember that if you compute a terminal value greater than book value, you are implying that the company will be able to maintain forever a return on capital employed in excess of its weighted average cost of capital. If you choose a lower value, you are implying that the company will enter a phase of decline after the explicit forecast period and that you think it will not be able to earn its cost of capital in the future. Lastly, if you assume that terminal value is equal to book value, you are implying that the company’s economic profit3 falls immediately to zero. This is the method of choice in the mining industry, for example, where we estimate a liquidation value by summing the scrap value of the various assets – land, buildings, equipment – less the costs of restoring the site.
In the case of ArcelorMittal, the capital employed end-2025 is \$53,266m, and discounted over five years at 10% results in \$33,074m. With this method, the enterprise value as at end-2020 becomes 16,071 + 33,074 = \$49,145m.
Generally speaking, no economic profit can be sustained forever. The company’s expected return on capital employed must gradually converge towards its cost of capital. This is the case with Coca-Cola, Michelin or British Airways. Regardless of the calculation method, the terminal value must reflect this. To model this phenomenon, we recommend using a “cash flow fade” methodology. In this approach, you define a time period during which the company’s return on capital employed diminishes, either because its margins shrink or because asset turnover declines. Ultimately, the ROCE falls down to the weighted average cost of capital. At the end of this time period, the enterprise value is equal to the book value of capital employed.
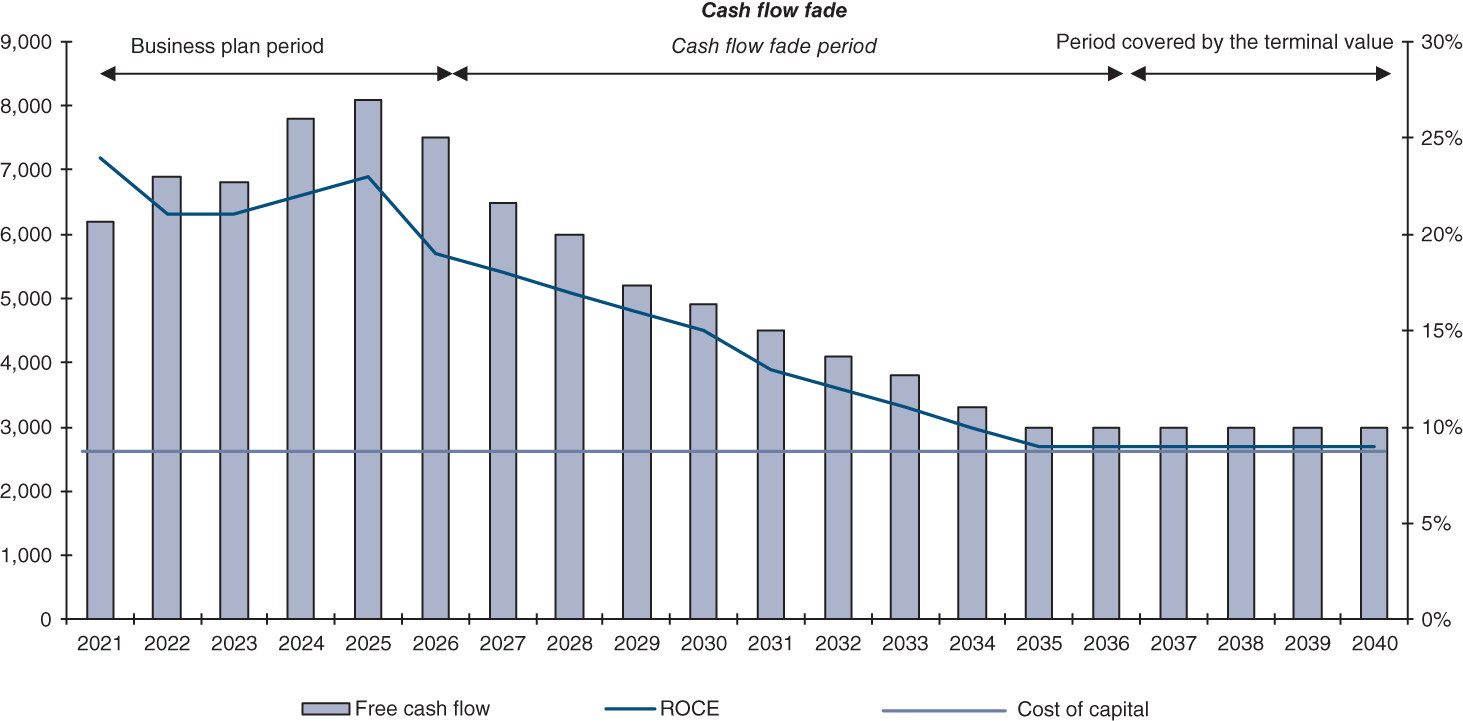
Readers will have to make choices: length of the cash flow fade period or speed of the convergence towards the cost of capital (form of the ROCE curve: convex, concave or a straight line as in our graph). They might also think that the company will be in a position to earn 1% or 2% more than its cost of capital due to the strength of its strategic position in its markets. Economic theory would not approve that!
This model can also be used for value-destroying companies. Sooner or later, there will be restructurings and bankruptcies triggering improvements in ROCE, but before applying the cash flow fade method the other way around, our readers would be well advised to ask themselves whether or not their company will be among the survivors!
2/ CHOOSING A DISCOUNT RATE
As we value cash flow to the firm, the discount rate is the weighted average cost of capital (WACC) or simply the cost of capital, which corresponds to the minimum rate of return required by the company’s fund providers, i.e. shareholders and lenders to finance the company’s capital employed. You may want to turn back to Chapter 29 for a more detailed look at this topic.
3/ THE VALUE OF NET DEBT
Once you obtain the enterprise value using the above methodology, you must remove the value of net debt taken at the date just before the start of the cashflows to be discounted (e.g. at 31 December 2020 for cashflows starting in 2021) to derive equity value. Net debt is composed of the value of all financial debt net of cash, i.e. of all bank borrowings, bonds, debentures and other financial instruments4 (short, medium or long-term), net of cash, cash equivalents and marketable securities.
Theoretically, the value of net debt is equal to the value of the future cash outflows (interest and principal payments) it represents, discounted at the market cost of similar borrowings. When all or part of the debt is listed or traded over the counter (listed bonds, syndicated loans), you can use the market value of the debt. You then subtract the market value of cash, cash equivalents and marketable securities.
The book value of net debt is often used as a first approximation of its present value. Nevertheless, in some cases, the value of debt can differ materially from its book value:
- when the firm has borrowed at fixed rates (directly after having swapped floating-rate debt) and rates have evolved since then;
- when the company’s solvency situation has significantly changed (for better or worse) since it has contracted the debt and there has been no spread adjustment to recognise this change;
- when the interest rate has been artificially reduced thanks to the issue of debt with warrants or other products (note that this would nevertheless be restated in IFRS or US GAAP accounts).
Hence, we strongly advise retaining the market value of debt rather than its book value when the net debt is high and the difference between book and market value is material.
For example, as at 31 December 2020, the book value of Rallye’s financial debt was €10,930m, its fair value was only €10,230m, a difference of €700m that represents twice the market capitalisation of Rallye as at mid-2021!
When the company’s business is seasonal, year-end working capital may not reflect average requirements, and debt on the balance sheet at the end of the year may not represent real funding needs over the course of the year (see Chapter 11). Some companies also perform year-end “window-dressing” in order to show a very low level of net debt. In these cases, if you notice that interest expense does not correspond to debt balances,5 you should restate the amount of debt by using a monthly average of outstanding net debt, for example.
Some other items may add complexity in the assessment of the real level of debt. For example, if assets have been removed from the balance sheet thanks to factoring or securitisation, they need to be added back in. In other cases, sellers may try to “dress” the balance sheet to show a level of debt lower than reality.
4/ OTHER VALUATION ELEMENTS
(a) Provisions
Provisions must only be included if cash flows exclude them. If the business plan’s EBIT does not reflect future charges for which provisions have been set aside – such as for restructuring, site closures, etc. – then the present value of the corresponding provisions on the balance sheet must be deducted from the value of the company after taxes (if the provision was not tax deductible).
Pension liabilities are a sticky problem (this is developed further in Chapter 7). How to handle them depends on how they were booked and, potentially, on the age pyramid of the company’s workforce. You will have to examine the business plan to see whether it takes future pension payments into account and whether or not a large group of employees is to retire just after the end of the explicit forecast period.
With rare exceptions, deferred tax liabilities generally remain relatively stable. In practice, they are rarely paid out. Consequently, they are usually not considered debt equivalents.
(b) Unconsolidated or equity-accounted investments
If unconsolidated or equity-accounted financial investments are not reflected in the projected cash flows (via dividends received), you should add their value to the value of discounted cash flows. In this case, use the market value of these assets including, if relevant, tax on capital gains and losses.
For listed securities, use listed market value. Conversely, for minor, unlisted holdings, the book value is often used as a shortcut. However, if the company holds a significant stake in the associated company – this is sometimes the case for holdings booked using the equity method – you will have to value the affiliate separately. This may be a simple exercise, applying, for example, a sector-average P/E to the company’s pro rata share of the net income of the affiliate. It can also be more detailed, by valuing the affiliate with a multi-criteria approach if the information is available.
(c) Tax-loss carryforwards
The value of any available tax loss carryforwards can be separately estimated by discounting the tax savings at the cost of capital until they are fully utilised. Alternatively, their use may be simulated in the calculation of free cash flow by using the standard tax rate only for the period following the exhaustion of the tax loss carryforwards.
(d) Minority interests
Future free cash flows calculated on the basis of consolidated financial information will belong partly to the shareholders of the parent company and partly to minority shareholders in subsidiary companies, if any.
This bias can be corrected by deducting minority interests from the enterprise value. If material, they should be valued separately and this can be done by performing a separate valuation of the subsidiaries in which some minority shareholders hold a stake. Naturally, this assumes you have access to detailed information about the subsidiaries.
You can also use a multiple approach. Simplifying to the extreme, you could apply the group’s implied P/E multiple to the minority shareholders’ portion of net profit to get a first-blush estimate of the value of minority interests. Alternatively, you could apply the group’s price-to-book ratio to the minority interests appearing on the balance sheet.
In either case, we would not recommend using book value to value minority interests unless amounts are low.
(e) Dilution
You might be wondering what to do with instruments that give future access to company equity, such as convertible bonds, warrants and stock options. If these instruments have a market value, your best bet will be to subtract that value from the enterprise value of the company to derive the value of equity capital, just as you would for net debt. The number of shares to use in determining the value per share will then be the number of shares currently in circulation.
Alternatively, you could use the treasury stock method (see Section 22.5) to measure the impact of these instruments on the value of the shares. Its drawback lies in ignoring the value of out-of-the money dilutive instruments.
5/ PROS AND CONS OF THE CASH FLOW APPROACH
The advantage of the discounted cash flow approach is that it quantifies the often implicit assumptions and projections of buyers and sellers. It also makes it easier to keep your feet closer to the ground during periods of market euphoria, excessively high valuations and astronomical multiples. It forces the valuation to be based on the company’s real economic performance.
You might be tempted to think this method works only to estimate the value of the majority shareholder’s stake and not for estimating the discounted value of a flow of dividends. You might even be tempted to go a step further and apply a minority discount to the present value of future cash flows for valuing a minority holding.
This is wrong. Applying a minority discount to the discounted cash flow method implies that you think the majority shareholder is not managing the company fairly. A discount is justified only if there are “losses in transmission” between free cash flow and dividends. This can be the case if the company’s strategy regarding dividends, borrowing and new investment is unsatisfactory.
Similarly, increasing the cash flow-based value can be justified only if the investor believes they can unlock synergies that will increase free cash flows.
To conclude, as satisfying as the DCF method is in theory, it presents three major drawbacks:
- It is very sensitive to assumptions and, consequently, the results it generates are very volatile. It is a rational method, but the difficulty in predicting the future brings significant uncertainty.
- It sometimes depends too much on the terminal value, in which case the problem is only shifted to a later period. Often the terminal value accounts for more than 50% of the value of the company, compromising the method’s validity. However, it is sometimes the only applicable method, such as in the case of a loss-making company for which multiples are inapplicable.
- Lastly, it is not always easy to produce projections over a sufficiently long period of time. External analysts may often find they lack critical information.
6/ DISCOUNTING CASH FLOW AND DISCOUNTING DIVIDENDS
Before people grew accustomed to using the discounted free cash flow to firm method,6 the dividend discount model was very popular: the value of a share is equal to the present value of all the cash flows that its owner is entitled to receive, namely the dividends, discounted at the cost of equity (kE).
This method is not an easy one to carry out if there is a regular change in the financial structure, which prompts a regular change in the cost of equity. But it is widely used to value banks, whose financial structures do not change much over time as a result of the capital adequacy ratios they must respect.7
This method is still used in very specific cases – for example, for companies in mature sectors with very good visibility and high payout ratios, such as utilities, concessions and real-estate companies.
Using the same logic, one can compute the value of equity by discounting free cash flow to equity (and no longer to firm) at the cost of equity. Free cash flow to equity is money available for shareholders, i.e. free cash flow to the firm minus after-tax interest payments and plus changes in net debt. We find this method quite theoretical and complex as the cost of equity and therefore the discount rate changes every year with the financial structure.
Section 31.3 MULTIPLE APPROACH OR PEER-GROUP COMPARISONS
1/ PRESENTATION
The peer comparison or multiples approach (or comparables, “comps” method) is based on three fundamental principles:
- the company is to be valued in its entirety;
- the company is valued at a multiple of its profit-generating capacity. The most commonly used are the P/E ratio, EBITDA and EBIT multiples;
- markets are efficient and comparisons are therefore justified.
Much like the DCF model, the approach is global, because it is based not on the value of individual operating assets and liabilities per se, but on the overall returns they are expected to generate. The value of the company is derived by applying a certain multiplier to the company’s profitability parameters. As we saw in Chapter 22, multiples depend on expected growth, risk and interest rates.
The approach is comparative. At a given point in time and in a given country, companies are bought and sold at a specific price level, represented, for example, by an EBIT multiple. These prices are based on internal parameters and by the overall stock market context. Prices paid for companies acquired in Europe in 2021, for example, when EBIT multiples were high (15 times on average) were not the same as for those acquired in 1980 when multiples hovered around four times EBIT.
Multiples can derive from a sample of comparable, listed companies or a sample of companies that have recently been sold. The latter sample has the virtue of representing actual transaction prices for the equity value of a company. These multiples are respectively called market multiples or trading multiples and transaction multiples. The first ones will be used to value shares of a firm that will not change control (for portfolio management or in the context of an IPO). The second ones are useful for assessing the value of equity in the context of change of control.
2/ BUILDING A SAMPLE OF COMPARABLE COMPANIES
For market multiples, a peer group comparison consists of setting up a sample of comparable listed companies that have not only similar sector and ideally geographic characteristics, but also similar operating characteristics, such as expected growth rates, size, etc. You should choose companies whose shares are liquid and are covered by a sufficient number of financial analysts.
3/ THE MENU OF MULTIPLES
There are two major groups of multiples: those based on the enterprise value (i.e. the value of capital employed), which is then used to obtain the value of equity, and those which are based directly on the value of equity.
Multiples based on the value of capital employed are multiples of operating balances before subtracting interest expense: EBIT and EBITDA multiples.
Multiples based on the value of equity are multiples of operating balances after interest expense, principally net income (P/E multiple), as well as multiples of cash flow and multiples of underlying income – i.e. before non-recurring items and the price book multiple (PBR).
(a) Multiples based on enterprise value
Whatever multiple you choose, you will have to value the capital employed for each listed company in the sample. This value is the sum of the company’s market capitalisation (or transaction value of equity for transaction multiples) and the value of its net debt at the valuation date and other adjustments presented.
In any case you need to be clear: the value of a minority stake will be deducted from the enterprise value if no dividend has been included in the company parameter (EBIT or EBITDA, which is normally the case), but not if it is included (net income). If pension assets minus pension liabilities have been added to enterprise value, then the part of pension cost corresponding to the interest cost shall not be included in the EBIT or EBITDA,8 etc.
You will then calculate the multiple for the comparable companies over three fiscal years: the current year, last year and next year. Note that we use the same value of capital employed in all three cases, as current market values should reflect anticipated changes in future operating results.9
Once the multiples of the comparable companies have been calculated and applied to the corresponding aggregates of the company to be valued, the value of the net debt and similar items presented on page 563 should be deducted from the value of the enterprise value arrived at in this way. The only case where the net debt included in these two valuation methods could be different is the subject of question 19 at the end of this chapter.
EBIT multiple
The EBIT multiple is the ratio of the enterprise value to EBIT (operating income). It takes into account the genuine profit-generating capacity of the sample companies.
You may have to perform some restatements in order to derive this operating income.
Consider the following sample of listed companies comparable to ArcelorMittal:
| €m | ThyssenKrupp | Voestalpine | Salzgitter | Nippon Steel | US Steel |
|---|---|---|---|---|---|
| Market capitalisation (value of equity) | 6,000 | 6,580 | 1,600 | 14,552 | 5,715 |
| + Value of debt | –3,782 | 3,281 | 879 | 17,135 | 2,565 |
| = Value of capital employed (A) | 2,218 | 9,087 | 2,479 | 31,687 | 8,280 |
| 2021e Operating income (EBIT) (B) | 424 | 782 | 366 | 4,104 | 1,848 |
| 2021e EBIT multiple (A/B) | 5.2 x | 11.6 x | 6.8 x | 7.7 x | 4.5 x |
The 2021 average pre-tax operating income (EBIT) multiple is 7.2 times. Applied to ArcelorMittal’s 2021e operating income of \$5,564m, comparable multiples would value ArcelorMittal’s enterprise value at \$40,584m and equity at \$26,584m, taking into account \$14,000m of debts.
EBITDA multiple
The EBITDA multiple follows the same logic as the EBIT multiple. It has the merit of eliminating the sometimes significant differences in depreciation methods and periods. It is very frequently used by stock market analysts for companies in capital-intensive industries which therefore record significant depreciation and amortisation charges.
Be careful when using the EBITDA multiple, however, especially when the sample and the company to be valued have widely disparate levels of margins. In these cases, the EBITDA multiple tends to overvalue companies with low margins and undervalue companies with high margins, independently of depreciation policy. Let’s take the following example:
| Group A | Group B | |
|---|---|---|
| Sales | 100 | 100 |
| EBITDA | 20 | 11 |
| Depreciation | 10 | 10 |
| EBIT | 10 | 1 |
| Enterprise value | 140 | ? |
Group A is valued at seven times its EBITDA. If we use this same multiple to value Group B, we derive an enterprise value for Group B of 77 (11 × 7). But if the cost structure of Group B remains the same in the future, its EBIT will always be close to 0; if that is the case, an investor would have difficulties paying a lot for such a company. The value of such a firm should be close to nil. This is the result we find if we prefer the EBIT multiple to the EBITDA multiple.
Other multiples
Operating multiples can also be calculated on the basis of other measures, such as turnover. Some industries have even more specific multiples, such as multiples of the number of subscribers, number of visitors or page views for Internet companies, tons of cement produced, etc. These multiples are particularly interesting when the return on capital employed of the companies in the sample is standard. Otherwise, results will be too widely dispersed. They are only meaningful for small businesses such as shops, where there are a lot of transactions and where, in many countries, turnover gives a better view of the profitability than the official profit figure.
These multiples are generally used to value companies that are not yet profitable: they were widely used during the Internet bubble, for instance. They tend to ascribe far too much value to the company to be valued, and we recommend that you avoid them.
(b) Multiples based on equity value
You may also decide to choose multiples based on operating balances after interest expense. These multiples include the P/E ratio, the cash flow multiple and the price-to-book ratio. All these multiples use market capitalisation at the valuation date (or price paid for the equity for transaction multiples) as their numerator. The denominators are net profit, cash flow and book equity, respectively. The net profit used by analysts is the company’s bottom line, i.e. the net profit attributable to the group (after deduction of minority interests) restated to exclude non-recurring items and the depreciation of goodwill, so as to put the emphasis on recurrent profit-generating capacity.
Using the same sample of comparable comparisons for ArcelorMittal presented before, we notice that the P/E ratio in 2021 for companies comparable to ArcelorMittal is 7.6:
| €m | ThyssenKrupp | Voestalpine | Salzgitter | Nippon Steel | US Steel |
|---|---|---|---|---|---|
| Market capitalisation (A) | 6,000 | 6,580 | 1,600 | 14,552 | 5,715 |
| 2017e Net income (B) | –278 | 528 | 241 | 1,820 | 1,652 |
| P/E ratio (A)/(B) | n.m. | 12.5 x | 6.6 x | 8.0 x | 3.5 x |
Applied to ArcelorMittal’s 2021e net income prediction of €3,790m, comparable multiples would value ArcelorMittal’s equity at €28,952m.
These multiples indirectly value the company’s financial structure, thus creating distortions depending on whether or not the companies in the sample are indebted.
Consider the following two similarly sized companies, Ann and Valeria, operating in the same sector and enjoying the same outlook for the future, with the following characteristics:
| Company | Ann | Valeria | |
|---|---|---|---|
| Operating income (EBIT) | 129 | 177 | |
| − | Interest expense | 9 | 120 |
| − | Corporate income tax (30%) | 36 | 17 |
| = | Net profit | 84 | 40 |
| Value of equity | 2,100 | ? | |
| Value of debt (at 3% and 10% p.a. respectively) | 300 | 1,200 |
Ann’s P/E ratio is 25 (2,100 / 84). As the two companies are comparable, we might be tempted to apply Ann’s P/E ratio to Valeria’s bottom line to obtain Valeria’s value of equity – i.e. 25 × 40 = 1,000.
Although it looks logical, this reasoning is flawed. Applying a P/E ratio of 25 to Valeria’s net income is tantamount to applying a P/E of 25 to Valeria’s NOPAT (177 × (1 – 30%) = 124) less a P/E of 25 applied to its after-tax interest expense (120 × (1 – 30%) = 84). After all, net income is equal to net operating profit after tax less interest expense after tax.
The first term (25 × NOPAT) should represent the enterprise value of Valeria, i.e. 25 × 124 = 3,100.
The second term (25 × after-tax interest expense) should thus represent the value of debt to be subtracted from enterprise value to give the value of equity capital that we are seeking. However, 25 × interest expense after tax is 2,100, whereas the value of the debt is only 1,200.
In this case, this type of reasoning would result in overstating the value of the debt (at 2,100 instead of 1,200) and then understating the value of the company’s equity.
The proper reasoning is as follows: we first use the multiple of Ann’s EBIT to get Valeria’s enterprise value. If Ann’s equity value is 2,100 and its debt is worth 300, then its enterprise value is 2,100 + 300, or 2,400. As Ann’s EBIT is 129, the multiple of Ann’s EBIT is 2,400 / 129 = 18.6. Valeria’s enterprise value is therefore equal to 18.6 times its EBIT, or 18.6 × 177 = 3,293. We now subtract the value of the debt (1,200) to obtain the value of equity capital, or 2,093. This is not the same as 1,000!
These distortions are the reason why financial analysts mostly use multiples of operating income (EBIT) or of operating income before depreciation and amortisation (EBITDA). This approach removes the bias introduced by different financial structures.
4/ TRANSACTION MULTIPLES
The approach is slightly different, but the method of calculation is the same. The sample is composed of information available on recent transactions in the same sector, such as the sale of a controlling block of shares, a merger, etc.
If we use the price paid by the acquirer, our multiple will contain the control premium the acquirer paid to obtain control of the target company. As such, the price includes the value of anticipated synergies. Using the listed share prices leads to a so-called minority value, which we now know is nothing other than the standalone value. In contrast, transaction multiples reflect majority value – i.e. the value including any control premium for synergies. For listed companies it has been empirically observed that control premiums are around 25% to 30% of pre-bid market prices (i.e. prices prior to the announcement of the tender offer).
You will find that it is often difficult to apply this method, because good information on truly comparable transactions is often lacking or incomplete (price paid not made public, unknown aggregates when the company is private, etc.).
As an example, the EBITDA multiples used in Europe for medium-sized company transfers are given in Section 31.6.
5/ MEDIANS, MEANS AND REGRESSIONS
People often ask if they should value a company by multiplying its profit-generating capacity by the mean or the median of the multiples of the sample of comparable companies. Our advice is to be wary of both means and medians, as they can mask wide disparities within the sample, and sometimes may contain extreme situations that should be excluded altogether. Try to understand why the differences exist in the first place rather than burying them in a mean or median value that has little real significance. For example, look at the multiples of the companies in the sample as a function of their expected growth. Sometimes this can be a very useful tool in positioning the company to be valued in the context of the sample.
Some analysts perform linear regressions to find a relationship between, for example, the EBIT multiple and expected growth in EBIT, the multiple of turnover and the operating margin, and the price-to-book ratio and the return on equity (to value banks).
This method allows us to position the company to be valued within the sample. The issue remaining, then, is to find the most relevant criterion. R2 indicates the significance of the regression line, and will be our guide in determining which criteria are the most relevant in the industry in question. Sometimes it allows you to choose a multiple outside the range of comparables’ multiples, simply because the company you are valuing has higher or lower expected growth than others you are comparing it with.
Section 31.4 THE SUM-OF-THE-PARTS METHOD (SOTP) OR NET ASSET VALUE (NAV)
The sum-of-the-parts method is simple. It consists in systematically studying the value of each asset and each liability on the company’s balance sheet. For a variety of reasons – accounting, tax, historical – book values are often far from reality. They must therefore be restated and revalued before they can be assumed to reflect a true net asset value. The sum-of-the-parts method is an additive method. Revalued assets are summed, and the total of revalued liabilities is subtracted.
For diversified groups, the SOTP or NAV method implies valuing subsidiaries or activities pro rata the ownership level using either the DCF or the multiples of comparable companies method. Then, the debt of the mother company10 is deducted as well as the present value of central costs. For example, UBS issued the following valuation in mid-2019 for Vivendi, a media group:
| Value | Valuation method | |
|---|---|---|
| Universal Music Group (80%) | 30,884 | Recent transaction |
| Canal+ (89%) | 4,896 | 2022 EBITDA multiple |
| Havas | 2,209 | 2022 EBITDA multiple |
| Editis | 663 | 2022 EBITDA multiple |
| Gameloft | 813 | Sales multiple |
| Vivendi Village | 47 | Sales multiple |
| Holding company and others | –1,423 | Cost multiple |
| Total enterprise value | 38,089 | |
| + Listed minority holdings | 3,513 | |
| incl. Telecom Italia (23.87%) | 1,594 | Stock market price |
| incl. Mediaset (28.8%) | 804 | Stock market price |
| inc. Lagardere (28.3%) | 835 | Stock market price |
| inc. Other (Prisa, Multichoice) | 279 | Stock market price |
| Non Listed minority holdings | 1,237 | |
| – Adjusted net debt (2021 Y/E) | –3,402 | |
| Equity value | 39,437 |
To apply this method properly, therefore, we must value each asset and each liability. Estimates must be consistent, even though the methods applied might be different.
Several basic types of value are used in the sum-of-the-parts method:
- market value: this is the value we could obtain by selling the asset. This value might seem indisputable from a theoretical point of view, but it virtually assumes that the buyer’s goal is liquidation. This is rarely the case. Acquisitions are usually motivated by the promise of industrial or commercial synergies;
- value in use: this is the value of an asset that is used in the company’s operations. It is a kind of market value at replacement cost;
- liquidation value: this is the value of an asset during a fire sale to get cash as soon as possible to avoid bankruptcy. It is market value minus a discount.
The sum-of-the-parts method is the easiest to use and the values it generates are the least questionable when the assets have a value on a market that is independent of the company’s operations, such as the property market, the market for aeroplanes, etc. It is hard to put a figure on a new factory in a new industrial estate. However, the value of the inventories and vineyards of a wine company is easy to determine and relatively undisputed, since a secondary market exists for these assets.
1/ TANGIBLE ASSETS
Tangible assets can be evaluated on the basis of replacement value, liquidation value, going-concern value or yet other values.
We do not intend to go into great detail here. Our main point is that in the sum-of-the-parts method it is important to determine an overall value for productive and commercial assets. Rather than trying to decompose assets into small units, you should reason on a general basis and consider sufficiently large groups of assets that have a standalone value (i.e. for which a market exists or that can operate on a standalone basis).
For example, it makes no sense to value the land on which a warehouse has been built. It makes more sense to value the combination of the land and the buildings on it. An appraiser will value the combination based on its potential productive capacity, not on the basis of its individual components. Of course, this is not the case if the objective is to reuse the land for something else, in which case you will want to deduct the cost of knocking down the warehouse.
2/ INVENTORIES
For industrial companies, valuing inventories usually does not pose a major problem, unless they contain products that are obsolete or in poor condition. In this case, we have to apply a discount to their book value, based on a routine inventory of the products.
In some situations, you will have to revalue the inventories of companies with long production cycles; the revaluation can lead to gains on inventories. This is often the case with champagne and cognac or even losses on completion not yet booked. The revaluations need to include their corporate income tax impact. In revaluing inventories, you must be aware that we are already buying the profits of the next few years.
3/ INTANGIBLE ASSETS
It might seem paradoxical to value intangible assets, since their liquidation value has, for a long time, been considered to be low. It is now widely acknowledged, however, that the value of a company is partly determined by the real value of its intangible assets, be they brand names, a geographical location or other advantages.
Some noteworthy examples:
- Brands: particularly hard to value but the importance of brands in valuation is growing.
In general, there are three methods for valuing brands:
- Method 1 The first method asks how much would have to be spent in advertising expense, after tax, to rebuild the brand. This method leads to undervaluation of new and successful brands (Netflix) and overvaluation of older and failing brands (the Amazon Fire phone).
- Method 2 The second method calculates the present value of all royalty payments that will or could be received from the use of the brand by a third party. It is very sensitive to the chosen royalty rate.
- Method 3 The third method consists in analysing the brand’s fundamental utility. After all, the brand’s raison d’être is to enable the company to sell more and at higher prices than would otherwise be possible without the brand name (costs of enhanced quality and services, of communication). Discounting this “excess profit” over a certain period of time should, after subtracting the related higher costs, yield an estimate of the value of the brand. Users of this method discount the incremental future operating income expected from the use of the brand and subtract the additional operating expense, working capital and investments, thereby isolating the value of the brand. We will not hide the fact that this approach, while intellectually appealing, is very difficult to apply in practice, because often there is no generic “control” product to use as a benchmark.
- Patents and technical know-how: they are valued as brands, but with the same difficulties.
- Lease rights: the present value of the difference between market rental rates and the rent paid by the company.
4/ TAX IMPLICATIONS
The acquirer’s objectives will influence the way taxes are included (or not) in the sum-of-the-parts approach.
- If the objective is to liquidate or break up the target company into component parts, then the acquirer will buy the assets directly, giving rise to capital gains or losses. The taxes (or tax credits) theoretically generated will then decrease (increase) the ultimate value of the asset.
- If the objective is to acquire some assets (and liabilities), and to run them as a going concern, then the assets will be revalued through the transaction. Increased depreciation will then lower income tax compared to liquidation or the breakup case above.11
- If the objective is to acquire a company and maintain it as a going concern (i.e. not discontinue its activities) and as a separate entity, then the acquiring company buys the shares of the target company rather than the underlying assets. It cannot revalue the assets on its books and will depreciate them from a lower base than if it had acquired the assets directly. As a result, depreciation expense will be lower and taxes higher.
5/ USEFULNESS OF SUM-OF-THE-PARTS VALUES
Sum-of-the-parts values can be deceptive, as many people think they imply safe or reliable values. In fact, when we say that a company has a high net asset value, it means that from a free cash flow point of view, the company’s terminal value is high compared with the value of intermediate cash flows. Consequently, the more “net asset value” a company has and the fewer cash flows it has, the more speculative and volatile its value is. Granted, its industrial risk may be lower, but most of the value derives from speculation about resale prices.
For this reason, the sum-of-the-parts method is useful for valuing small companies with no particular strategic value, or companies whose assets can be sold readily on a secondary market (aeroplanes, cinemas, etc.).
Section 31.5 COMPARISON OF VALUATION METHODS
1/ RECONCILING THE DIFFERENT METHODS OF VALUATION
If markets are efficient, then all of the valuation methods discussed so far should lead to the same valuation. In reality, however, there are often differences among the sum-of-the-parts value, the DCF-based value and the peer-comparison value. You must analyse the source of these differences and resist the temptation to average them!
(a) Analysing the difference between sum-of-the-parts value and discounted cash flow value
If the sum-of-the-parts value is higher than the DCF value or the value derived from a comparison of multiples (both return based values), then the company is being valued more for its past, its revalued equity capital, than for its outlook for future profitability. In this case, the company should not invest but divest, liquidating its assets to boost profitability and improve the allocation of its resources. This is often the punishment for overly complacent management.
This strategy occurs at regular intervals (in the 1980s, in 2007–2008). Companies were bought up on the open market, and then sold off piecemeal. The buyer realises a gain because the parts are worth more than the company as a whole. Alternatively, often under pressure from activist investors (Chapter 41), the company can of its own volition embark on this refocusing process (General Electric, ThyssenKrupp, Seimens).
If the sum-of-the-parts value is lower than the DCF value or the value derived from multiples, which is the usual case in an economy where companies have a lot of intangibles, then the company is very profitable and invests in projects with expected profitability greater than their cost of capital. The company has real expertise, strong strategic positioning and enjoys high barriers to entry. But the chances are that it will not escape competitive pressure forever.
Goodwill value has long been used to correct the restated net asset value to take into account the anticipated return on capital employed of the firm compared to its cost of capital and hence to value its “intangible capital”.
The starting point of all these mixed methods was to determine the capital employed, restated for potential capital gains or losses. Then a normative operating profit was computed by applying the cost of capital to the capital employed. The difference between the actual operating profit and the normative operating profit was called super-profit (leading to goodwill if positive and badwill if negative). The super-profit is to be discounted over a certain period to derive the value of goodwill. This is conceptually close to the EVA.
(b) Comparison values versus DCF values
If the value obtained via peer comparison is greater than the DCF-based value (and if all the calculations are correct!), then the company’s managers should be thinking about floating the company on the stock exchange, because financial investors have a more positive view of the company’s risk profile and profitability outlook than its management or current shareholders. Conversely, if the value obtained by comparison is lower than the DCF value and if the business plan is reliable, it would be wiser to wait until more of the long-term growth potential in the company’s business plan feeds through to its financial statements before launching an IPO; and perhaps do a public-to-private (see Section 47.2, 2/) if the company is already listed.
If transaction multiples generate a significantly higher value than market multiples or the DCF model, then it would be better to organise a trade sale by soliciting bids from several industry participants. In short, look before you leap!
(c) Is there one valuation method for selling a company and another for buying it?
There is no technical reason why a seller should not use one valuation method and the buyer another:
- A seller usually favours the DCF method as it is based upon a business plan which is rarely built on pessimistic assumptions! Most business plans are established by the management under instruction from selling shareholders. But in the back of their mind, a seller will not forget results obtained with a peer-comparison method, as they will be very reluctant to sell at a lower multiple than the one obtained by a competitor a few months ago or the one they could get through an IPO.
- A buyer will use the peer-comparison method to justify a lower price than that resulting from the DCF. They will claim that other buyers have paid 100 and there is no reason why they should pay 120 or 130. Nevertheless, at the back of their mind the buyer is thinking about their own business plan, including synergies and new developments. They will soon be able to compute their own DCF to check whether the price they will pay is expected to create value for their own shareholders.
2/ THE LIFECYCLE THEORY OF COMPANY VALUE
Companies that have achieved a certain level of success will see their sum-of-the-parts and cash flow values differ throughout their lifecycle. Lifecycle is an important factor in determining the value of companies, as it was in determining the optimal capital structure and financing policies.
When the company is founded, its net asset value and cash flow value are identical; the company has not yet made any investments. After the first year or two of operations, net asset value may dip because of start-up losses. Meanwhile, cash flow value is greater because it anticipates hopefully positive future profitability.
During the growth phase, net asset value will rise as all or part of the company’s profits are reinvested and the company builds a customer base (the value of which does not appear in the accounts, however). Cash flow value also continues to rise and remains above the net asset value. The company’s expertise has not yet become a tangible asset. It is still associated with the people who developed it.
At maturity, cash flow value will start growing more slowly or stop growing altogether, reflecting a normal profit trend. Nonetheless, the net asset value continues to grow, but more slowly because the company increases its payout ratio. Broadly speaking, net asset value and cash flow value are very close.
If the company then enters a phase of decline, its profits decline and the cash flow value slips below net asset value. The latter continues to grow but only very slowly, until the company starts posting losses. The net asset value falls. As for cash flow value, it drops very low. The net asset value then becomes particularly speculative.
At any given point in time, it is very important to understand the reasons for the difference between the net asset value and the cash flow value, because this understanding gives important clues as to the situation and future prospects of the company.
You might now be thinking that our kaleidoscope of methods leads to as many values as there are images of the company:
- sum-of-the-parts, or net asset value;
- peer-comparison value;
- intrinsic value (i.e. DCF), etc.
We advise against calculating a wide variety of valuations, unless it is to show that you can prove anything when it comes to valuation. But you must not throw up your hands in despair either. Instead, try to understand each type of value, which corporate circumstances it applies to and what its implicit assumptions are. It is more important to determine ranges than to come up with precise values. Precision is the domain of negotiation, the goal of which is to arrive at an agreed price.
Lastly, remember that valuing a company means:
- taking a speculative stance not only on the future of the company, but also on its market conditions. The cash flow and comparison methods demonstrate this;
- implicitly extrapolating past results or expected near-term results far into the future, opening the door to exaggeration;
- sometimes forgetting that net asset value is not a good reference if the profitability of the company differs significantly from its investors’ required return.
Shareholders’ decisions to sell all or part of a company are based on the price they believe they can obtain compared to their set of calculated valuations.
Section 31.6 PREMIUMS AND DISCOUNTS
A newcomer to finance might think that the market for the purchase and sale of companies is a separate market with its own rules, its own equilibria, its own valuation methods and its own participants.
This is absolutely wrong. Indeed, the market for corporate control is simply a segment of the financial market. The valuation methods used in this segment are based on the same principles as those used to measure the value of a financial instrument. Experience has proven that the higher the stock market, the higher the price of unlisted companies, as the following graphic illustrates:

Source: Argos Wityu and Facset
Participants in the market for corporate control think the same way as investors in the financial market. Of course, the smaller the company is, the more tenuous is the link. The value of a butcher’s shop or a bakery is largely intangible and hard to measure, and thus has little in common with financial market values. But in reality, only appearances make the market for corporate control seem fundamentally different.
1/ STRATEGIC VALUE AND CONTROL PREMIUM
There is no real control value other than strategic value, which we will develop later. Gone are the days when the control premium was reserved for the majority shareholder and minority shareholders were deprived of it. Stock markets have established equal treatment of shareholders as an absolute principle: minority shareholders are entitled to the same transfer price as the majority shareholder receives for their controlling stake.
Shareholder agreements are a common method for expressing this principle in unlisted companies.
Nevertheless, entrepreneursoften have a diametrically opposed view. For them, minority shareholders are passive beneficiaries of the fruits of all the personal energy the managers/majority shareholders have invested in the company. It is difficult to convince entrepreneurs that the roles of management and shareholders can be separated and that they must be compensated differently – and especially that the risk assumed by all types of shareholders must be rewarded.
If we assume that markets are efficient, then the existence of such a premium can be justified only if the new owners of the company obtain more value from it than its previous owners did. A control premium derives from the industrial, commercial, administrative or tax synergies the new majority shareholders hope to unlock. They hope to improve the acquired company’s results by managing it better, pooling resources, combining businesses or taking advantage of economies of scale. These value-creating actions are reflected in the buyer’s valuation. The trade buyer (i.e. an acquirer who already has industrial operations) wants to acquire the company so as to change the way it is run and, in doing so, create value.
The company is therefore worth more to a trade buyer than it is to a financial buyer, who values the company on a standalone basis, as one investment opportunity among others, independently of these synergies.
In this light, we now understand that the trade buyer’s expectations are not the same as those of the financial investor. This difference can lead to a different valuation of the company. We call this strategic value.
Strategic value is the maximum value a trade buyer is prepared to pay for a company. It includes the value of projected free cash flows of the target on a standalone basis, plus the value of synergies from combining the company’s businesses with those of the trade buyer. It also includes the value of expected improvement in the company’s profitability compared to the business plan provided, if any.
We previously demonstrated that the value of a financial security is independent of the portfolio to which it belongs, but now we are confronted with an exception. Depending on whether a company belongs to one group of companies or another, it does not have the same value. Make sure you understand why this is the case. The difference in value derives from different cash flow projections, not from a difference in the discount rate applied to them, which is a characteristic of the company and identical for all investors. The principles of value are the same for everyone, but strategic value is different for each trade buyer, because each of them places a different value on the synergies they believe it can unlock and on their ability to manage the business better than current management.
As the seller will also hope to benefit from the synergies, negotiation will focus on how the additional profitability the synergies are expected to generate will be shared between the buyer and the seller.
But some industrial groups go overboard, buying companies at twice their standalone value on the pretext that their strategic value is high or that establishing a presence in such-and-such geographic location is crucial. They are in for a rude awakening. Sometimes the market has already put a high price tag on the target company. Specifically, when the market anticipates merger synergies, speculation can drive the share price far above the company’s strategic value, even if all synergies are realised. In other cases, a well-managed company may benefit little or even be hurt by teaming up with another company in the same industry, meaning either that there are no synergies to begin with or, worse, that they are negative, or that the absorption of the company into a much larger group demotivates its managers.
2/ NEGATIVE CONTROL VALUE
Groups can sometimes sell, at a negative price, subsidiaries that are heavily loss-making and that they are unable to turn around. That is, they pay a buyer a sum of money, so that the buyer agrees to buy the shares of that subsidiary from them for a symbolic euro. Thus, in these atypical cases, there is a negative control premium! Materially, the group subscribes to a capital increase of its subsidiary until it reduces its net indebtedness to the negative price agreed with the buyer. Then the transfer of control takes place for one euro.
As the transfer price represents one to three years of losses, from the seller’s point of view they have concluded a good financial transaction. Indeed, at a constant loss, the seller recovers their final investment in one to three years, which is a very short payback period. They do not have to carry out a major restructuring or liquidation, which would be damaging to their reputation, especially if they are successful in other sectors.
Following the acquisition for one euro, the buyer will find a net cash position that will enable them to finance part of the company’s recovery.
This negative price scenario obviously does not concern listed companies. Thus, in 2021 Saint Gobain sold Lapeyre, which was highly loss-making, to the German turnaround fund Mutares for a negative price of €243m, i.e. 3 times 2020 losses.
3/ MINORITY DISCOUNTS AND PREMIUMS
We have often seen minority holdings valued with a discount, and you will quickly understand why we believe this is unjustified. A “minority discount” would imply that minority shareholders have proportionally less of a claim on the cash flows generated by the company than the majority shareholder. This is not true.
In fact, a shareholder who already has the majority of a company’s shares may be forced to pay a premium to buy the shares held by minority shareholders. On average, in Europe, the premium paid to buy out minorities is in the region of 10 to 20% (but the premium can be significantly higher for low liquidity listed companies), less than that paid to obtain control. Indeed, majority shareholders may be willing to pay such a premium if they need full control over the acquired company to implement certain synergies.
Having said that, the lack of liquidity associated with certain minority holdings, either because the company is not listed or because trading volumes are low compared with the size of the minority stake, can justify a discount. In this case, the discount does not really derive from the minority stake per se, but from its lack of liquidity. A minority shareholder, aware of the value of the shareholding it holds, will only be able to sell it if the majority shareholder also decides to sell.
Lack of liquidity may increase the volatility of the share price. Therefore, investors will discount an illiquid investment at a higher rate than a liquid one. The difference in values results in a liquidity discount.
We have encountered some cases where the liquidity discount exceeded 50% for a minority shareholder that wanted to sell its shares, which the majority shareholder only offered to buy after three years of discouraging all potential acquirers. But we have also seen the lack of discount when the disposal of a small stake could change the balance of power in a company.
This is similar to the situation of a listed company with a reduced free float, where the minority shareholder is then in the hands of the majority shareholder who controls the market communication of the firm. Some listed firms can suffer from an undervaluation due to reduced liquidity of the share, so analysts do not publish research and it then becomes a vicious circle.
In a listed company of sufficient size with widely spread capital, the situation is different as the minority shareholder will be protected by the relevant share price and the protection afforded by market authorities.
SUMMARY
QUESTIONS
EXERCISES
ANSWERS
BIBLIOGRAPHY
NOTES
- 1 Earnings before interest, taxes, depreciation and amortisation.
- 2 All the more so as in mature sectors inflation is lower than in the economy in general.
- 3 NOPAT (EBIT after tax) – WACC × Capital employed.
- 4 Including the value of hedging instruments, if any.
- 5 The interest rate calculated as interest in the income statement/net debt in the closing balance sheet does not reflect the actual interest rates paid on the ongoing debt during the year.
- 6 That is, before 1995 in Europe and the US.
- 7 Basel III and IV.
- 8 Which could be the case under IFRS.
- 9 For more on this, see the Vernimmen.com Newsletter no. 24, May 2007.
- 10 Not that of subsidiaries, as it has already been taken into account when valuing the subsidiaries.
- 11 Acquisition of assets will most often generate deductible depreciation whereas acquisition of shares of a company will generate goodwill, which in most European countries does not give rise to tax-deductible amortisation.
